Modeling air entrainment and transport in a hydraulic jump using two-fluid RANS and DES turbulence models | Heat and Mass Transfer Skip to main content
Springer Nature Link
Log in

Modeling air entrainment and transport in a hydraulic jump using two-fluid RANS and DES turbulence models

  • Original
  • Published:
Heat and Mass Transfer Aims and scope Submit manuscript

Abstract

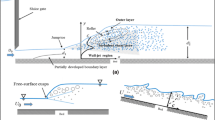
Both RaNS (Reynolds-averaged Navier–Stokes) and DES (Detached Eddy Simulation) type turbulence models were used in conjunction with a two-fluid model of bubbly flow and a new subgrid air entrainment model to predict air entrainment and transport in a hydraulic jump. It was found that the void fraction profiles predicted by both methods are in agreement with the experimental data in the lower shear layer region, which contains the air bubbles entrained at the so-called toe of the hydraulic jump. In contrast, in the upper roller region behind the toe, the averaged results of the DES turbulence model gives accurate predictions while a RaNS turbulence model does not. This is because the DES turbulence model successfully captures the strong fluctuations on the free surface which allows it to entrain air near the top of the roller region. In contrast, RaNS type turbulence model results in a steady, smooth interface which fails to capture the wave-induced bubble sources in that region. To our knowledge, this study is the first successful quantitative numerical simulation of the overall void fraction profiles in a hydraulic jump.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

References

  1. Kalinske A, Robertson J (1943) Closed conduit flow. Trans ASCE 108:1435–1447

    Google Scholar 

  2. Rajaratnam N (1962) An experimental study of air entrainment characteristics of the hydraulic jump. J Inst Eng India 42(7):247–273

    Google Scholar 

  3. Resch F, Leutheusser H (1972) Le ressaut hydraulique: mesure de turbulence dans la rgion diphasique. Jl La Houille Blanche 4:279–293

    Article  Google Scholar 

  4. Chanson H (1996) Air bubble entrainment in free-surface turbulent shear flows. Academic Press, Massachusetts

    Google Scholar 

  5. Mossa M, Tolve U (1998) Flow visualization in bubbly two-phase hydraulic jump. J Fluids Eng 120(1):160–165

    Article  Google Scholar 

  6. Chanson H, Brattberg T (2000) Experimental study of the air-water shear flow in a hydraulic jump. Int J Multiph Flow 26(4):583–607

    Article  MATH  Google Scholar 

  7. Murzyn F, Mouaze D, Chaplin J (2005) Optical fibre probe measurements of bubbly flow in hydraulic jumps. Int J Multiph Flow 31(1):141–154

    Article  MATH  Google Scholar 

  8. Liu Q, Uwe D (1994) Turbulence characteristics in free and forced hydraulic jumps. J Hydraulic Res 32:877–898

    Article  Google Scholar 

  9. Stelling GS, Busnelli MM (2001) Numerical simulation of the vertical structure of discontinuous flows. Int J Numer Methods Fluids 37(1):23–43

    Article  MATH  Google Scholar 

  10. Zhou J, Stansby P (1999) 2d shallow water flow model for the hydraulic jump. Int J Numer Methods Fluids 29(4):375–387

    Article  MATH  Google Scholar 

  11. Ma J, Oberai A, Drew D, Lahey Jr. R, Hyman M (2009) A comprehensive subgrid air entrainment model for Reynolds-averaged simulations of free-surface bubbly flows. In: APS DFD meeting abstracts

  12. Ma J, Oberai A, Drew D, Lahey R Jr, Hyman M (2011) A comprehensive sub-grid air entrainment model for RaNS modeling of bubbly flows near the free surface. J Comput Multiph Flows 3(1):41–56

    Article  Google Scholar 

  13. Souders DT, Hirt CW (2004) Modeling entrainment of air at turbulent free surfaces. In: Proceedings of world water and environmental resources congress 2004

  14. Gonzlez AE, Bombardelli FA (2005) Two-phase-flow theoretical and numerical models for hydraulic jumps, including air entrainment. In: Proceedings of XXXI IAHR congress

  15. Moraga FJ, Carrica PM, Drew DA, Lahey RT Jr (2008) A sub-grid air entrainment model for breaking bow waves and naval surface ships. Comput Fluids 37(3):281–298

    Article  Google Scholar 

  16. Martinez-Bazan C, Montañes J, Lasheras JC (1999) On the breakup of an air bubble injected into a fully developed turbulent flow. Part 1. Breakup frequency. J Fluid Mech 401:157–182

    Article  MATH  Google Scholar 

  17. Larreteguy AE, Drew DA, Lahey Jr. RT (2002) A center-averaged two-fluid model for wall-bounded bubbly flows. In: Proceedings of 2002 joint US ASME/European fluids engineering division summer meeting, Montreal

  18. Moraga FJ, Larreteguy AE, Drew DA, Lahey RT Jr (2006) A center-averaged two-fluid model for wall-bounded bubbly flows. Comput Fluids 35:429–461

    Article  MATH  Google Scholar 

  19. Sussman M, Smereka P, Osher S (1994) A level set approach for computing solutions to incompressible two-phase flow. J Comput Phys 114(1):146–159

    Article  MATH  Google Scholar 

  20. Carrica P, Wilson R, Stern F (2006) An unsteady single-phase level set method for viscous free surface flows. Int J Numer Methods Fluids 53(2):229–256

    Article  MathSciNet  Google Scholar 

  21. Menter FR (1994) Two-equation eddy viscosity turbulence models for engineering applications. AIAA J 32(8):1598–1605

    Article  Google Scholar 

  22. Paterson EG, Wilson RV, Stern F (2003) General-purpose parallel unsteady rans ship hydrodynamics code: Cfdship-Iowa. IIHR Report 432, Iowa Institute for Hydraulic Research. The University of Iowa, Iowa

    Google Scholar 

  23. Travin A, Shur M, Strelets M, Spalart PR (2002) Physical and numerical upgrades in the detached-eddy simulation of complex turbulent flows. Springer, Netherlands, pp 239–254

    Google Scholar 

  24. Moraga FJ, Larreteguy AE, Carrica PM, Drew DA, Lahey Jr. RT (2001) CFDShipM: multiphase code for ship hydrodynamics. Version 3.02.02 users manual

  25. Misra SK, Brocchini M, Kirby JT (2006) Turbulent interfacial boundary conditions for spilling breakers. In: Proceedings of the 30th international conference of coastal engineering

  26. Rodriguez-Rodriguez J, Marugan-Cruz C, Aliseda A, Lasheras JC (2011) Dynamics of large turbulent structures in a steady breaker. Exp Thermal Fluid Sci 35:301–310

    Article  Google Scholar 

Download references

Acknowledgments

This work was supported by the Office of Naval Research (ONR), Grant N00014-03-1-0826, under the administration of Dr. Patrick Purtell, and was also supported in part by a generous grants of computer time from the DOD High Performance Computing Modernization Program at the Maui High Performance Computing Center (MHPCC), US Army Engineering and Research Development Center (ERDC) and Arctic Region Supercomputing Center (ARSC). We also thank Dr. Frederic Murzyn at Parc Universitaire de Laval-Change for providing us with his detailed experimental data on void fractions in hydraulic jumps.

Author information

Authors and Affiliations

  1. Center for Multiphase Research, Rensselaer Polytechnic Institute, 110 8th St., Troy, NY, 12180-3590, USA

    Jingsen Ma, Assad A. Oberai, Richard T. Lahey Jr & Donald A. Drew

Authors
  1. Jingsen Ma
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Assad A. Oberai
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Richard T. Lahey Jr
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Donald A. Drew
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Assad A. Oberai.

Additional information

Dedicated to Prof. Dr.-Ing. Dr.-Ing. E.h. mult. Franz Mayinger on the occasion of his 80th birthday.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Ma, J., Oberai, A.A., Lahey, R.T. et al. Modeling air entrainment and transport in a hydraulic jump using two-fluid RANS and DES turbulence models. Heat Mass Transfer 47, 911–919 (2011). https://doi.org/10.1007/s00231-011-0867-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00231-011-0867-8

Keywords

Search

Navigation