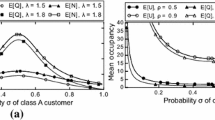
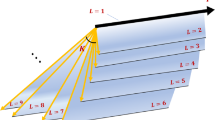
Abstract
Whereas the buffer content of batch-service queueing systems has been studied extensively, the customer delay has only occasionally been studied. The few papers concerning the customer delay share the common feature that only the moments are calculated explicitly. In addition, none of these surveys consider models including the combination of batch arrivals and a server operating under the full-batch service policy (the server waits to initiate service until he can serve at full capacity). In this paper, we aim for a complete characterisation—i.e., moments and tail probabilities - of the customer delay in a discrete-time queueing system with batch arrivals and a batch server adopting the full-batch service policy. In addition, we demonstrate that the distribution of the number of customer arrivals in an arbitrary slot has a significant impact on the moments and the tail probabilities of the customer delay.
Similar content being viewed by others
References
Arumuganathan R, Jeyakumar S (2005) Steady state analysis of a bulk queue with multiple vacations, setup times with N-policy and closedown times. Appl Math Model 29: 972–986
Bailey NTJ (1954) On queueing processes with bulk service. J R Stat Soc 16(1): 80–87
Bruneel H, Kim BG (1993) Discrete-time models for communication systems including ATM. Kluwer, Boston
Bruneel H, Steyaert B, Desmet E, Petit GH (1994) Analytic derivation of tail probabilities for queue lengths and waiting times in ATM multiserver queues. Eur J Oper Res 76: 563–572
Chang SH, Choi DW (2005) Performance analysis of a finite-buffer discrete-time queue with bulk arrival, bulk service and vacations. Comp Oper Res 32: 2213–2234
Chang SH, Takine T (2005) Factorization and stochastic decomposition properties in bulk queues with generalized vacations. Queueing Syst 50: 165–183
Chaudhry ML, Templeton JGC (1983) A first course in bulk queues. John Wiley & Sons, New York
Claeys D, Walraevens J, Laevens K, Bruneel H (2007) A discrete-time queueing model with a batch server operating under the minimum batch size rule. In: Proceedings of the 7th international conference on next generation teletraffic and wired/wireless advanced networking (NEW2AN 2007), St. Petersburg, September 10–14, pp 248–259
Claeys D, Laevens K, Walraevens J, Bruneel H (2008) Delay in a discrete-time queueing model with batch arrivals and batch services. In: Proceedings of the fifth international conference on information technology: new generations (ITNG 2008), Las Vegas, Nevada, April 7–9, pp 1040–1045
Cohen JW (1969) The single server queue. North-Holland, Amsterdam; Wiley Interscience, New York
Dagsvik J (1975) The general bulk queue as a matrix factorisation problem of the Wiener-Hopf type. Part 1. Adv Appl Prob 7(3): 636–646
Downton F (1955) Waiting time in bulk service queues. J R Stat Soc Ser B (Methodol) 17(2): 256–261
Dümmler MA, Schömig AK (1999) Using discrete-time analysis in the performance evaluation of manufacturing systems. In: Proceedings of the 1999 international conference on semiconductor manufacturing operational modeling and simulation (SMOMS ’99), San Francisco, California, January 18–20
Fiems D, Bruneel H (2002) A note on the discretization of Little’s result. Oper Res Lett 30: 17–18
Goswami V, Mohanty JR, Samanta SK (2006) Discrete-time bulk-service queues with accessible and non-accessible batches. Appl Math Comput 182: 898–906
Gupta UC, Goswami V (2002) Performance analysis of finite buffer discrete-time queue with bulk service. Comp Oper Res 29: 1331–1341
Keilson J (1962) The general bulk queue as a Hilbert problem. J R Stat Soc Ser B (Methodol) 24(2): 344–358
Kim NK, Chaudhry ML (2006) Equivalences of batch-service queues and multi-server queues and their complete simple solutions in terms of roots. Stoch Anal Appl 24: 753–766
Lee HW, Lee SS, Chae KC (1996) A fixed-size batch service queue with vacations. J Appl Math Stoch Anal 9: 205–219
Medhi J (1975) Waiting time distributions in a Poisson queue with a general bulk service rule. Manage Sci 21(2): 777–782
Miller RG (1959) A contribution to the theory of bulk queues. J R Stat Soc Ser B (Methodol) 21(2): 320–337
Neuts MF (1967) A general class of bulk queues with Poisson input. Ann Math Stat 38: 759–770
Powell WB, Humblet P (1986) The bulk service queue with a general control strategy: theoretical analysis and a new computational procedure. Oper Res 34(2): 267–275
Samanta SK, Chaudhry ML, Gupta UC (2007) Discrete-time Geo X|G (a,b)|1|N queues with single and multiple vacations. Math Comp Model 45: 93–108
Sikdar K, Gupta UC (2005) Analytic and numerical aspects of batch service queues with single vacation. Comp Oper Res 32: 943–966
Yi XW, Kim NK, Yoon BK, Chae KC (2007) Analysis of the queue-length distribution for the discrete-time batch-service Geo X|G a,Y|1|K queue. Eur J Oper Res 181: 787–792
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Claeys, D., Laevens, K., Walraevens, J. et al. Complete characterisation of the customer delay in a queueing system with batch arrivals and batch service. Math Meth Oper Res 72, 1–23 (2010). https://doi.org/10.1007/s00186-009-0297-2
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00186-009-0297-2