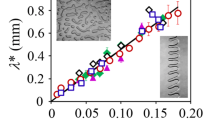
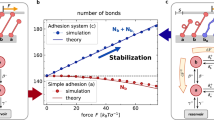
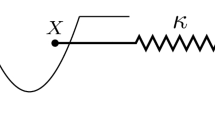Abstract
We study the mechanics of a reversible decohesion (unzipping) of an elastic layer subjected to quasi-static end-point loading. At the micro level the system is simulated by an elastic chain of particles interacting with a rigid foundation through breakable springs. Such system can be viewed as prototypical for the description of a wide range of phenomena from peeling of polymeric tapes, to rolling of cells, working of Gecko’s fibrillar structures and denaturation of DNA. We construct a rigorous continuum limit of the discrete model which captures both stable and metastable configurations and present a detailed parametric study of the interplay between elastic and cohesive interactions. We show that the model reproduces the experimentally observed abrupt transition from an incremental evolution of the adhesion front to a sudden complete decohesion of a macroscopic segment of the adhesion layer. As the microscopic parameters vary the macroscopic response changes from quasi-ductile to quasi-brittle, with corresponding decrease in the size of the adhesion hysteresis. At the micro-scale this corresponds to a transition from a ‘localized’ to a ‘diffuse’ structure of the decohesion front (domain wall). We obtain an explicit expression for the critical debonding threshold in the limit when the internal length scales are much smaller than the size of the system. The achieved parametric control of the microscopic mechanism can be used in the design of new biological inspired adhesion devices and machines.
Similar content being viewed by others
References
Braides, A.: Gamma-Convergence for Beginners. Oxford University Press, Oxford (2002)
Braun O.M., Kivshar S.: The Frenkel–Kontorova model. Springer, Berlin (2003)
Bucur, D., Buttazzo, G.: Variational methods in shape optimization problems. In: Progress in Nonlinear Differential Equations and Their Applications. Birkhäuser, Boston (2005)
Chen S., Gao H.: Bio-inspired mechanics of reversible adhesion:orientation dependent adhesion strength for non-slipping adhesive contact with transversely isotropic elastic materials. J. Mech. Phys. Solids 55, 1001–1015 (2007)
Dal Maso G.: An Introduction to Γ-Convergence. Birkhäuser, Boston (1993)
Del Piero G., Truskinovsky L.: Elastic bars with cohesive energy. Cont. Mech. Thermodyn. 21(2), 141–171 (2009)
Dembo M., Torney D.C., Saxman K., Hammer D.: The reaction-limited kinetics of membrane-to-surface adhesion and detachment. Proc. R. Soc. Lond. B 234, 55–83 (1988)
Deshpande V.S., Mrksich M., McMeeking R.M., Evans A.G.: A bio-mechanical model for coupling cell contractility with focal adhesion formation. J. Mech. Phys. Sol. 56, 1484–1510 (2008)
Frémond M.: Contact with Adhesion, Chap. 4. Birkhäuser, Verlag (1988)
Geim A.K., Dubonos S.V., Grigorieva I.V., Novoselov K.S., Zhukov A.A., Shapoval S.Y.: Microfabricated adhesive mimicking gecko foot-hair. Nat. Mater. 2, 461–463 (2003)
Hu G.Y., Connell R.F.: Analytic inversion of symmetric tridiagonal matrices. J. Phys. A 29, 1511–1513 (1996)
Jagota A., Bennison S.J.: Mechanics of adhesion through a fibrillar microstructure. Integr. Comp. Biol. 42, 1140–1145 (2002)
Kendall K.: Molecular Adhesion and its Applications. Kluwer, Dordrecht (2004)
Kresse O., Truskinovsky L.: Lattice friction for crystalline defects: from dislocations to cracks. J. Mech. Phys. Solids 52, 2521–2543 (2003)
Lipowsky R.: Critical effects at complete wetting. Phys. Rev. B 32, 1731 (1985)
Maddalena F., Percivale D.: Variational models for peeling problems. Interf. Free Bound. 10, 503–516 (2008)
Marigo J.J., Truskinovsky L.: Initiation and propagation of fracture in the models of Griffith and Barenblatt. Continuum Mech. Thermdyn. 16, 391–409 (2004)
Mefti N., Haussy B., Ganghoffer J.F.: Mechanical modeling of the rolling phenomenon at the cell scale. Int. J. Solids Struct. 43, 7378–7392 (2006)
Nabben R.: Two-sided bounds on the inverses of diagonally dominant tridiagonal matrices. Linear Algebra Appl. 287, 289–305 (1999)
Oyharcabal X., Frisch T.: Peeling off an elastica from a smooth attractive substrate. Phys. Rev. E 71, 0366111–0366116 (2005)
Persson B.N.J.: On the mechanism of adhesion in biological systems. J. Chem. Phys 118, 7614–7621 (2003)
Peyrard M.: Nonlinear dynamics and statistical physics of DNA. Nonlinearity 17, R1–R40 (2004)
Podio-Guidugli P.: Peeling tapes. In: Steinmann, P., Maugin, G. (eds) Mechanics of Material Forces, vol. 13., Springer, Heidelberg (2005)
Puglisi G., Truskinovsky L.: Rate independent hysteresis in a bi-stable chain. J. Mech. Phys. Solids 50(2), 165–187 (2002)
Puglisi G., Truskinovsky L.: Thermodynamics of rate independent plasticity. J. Mech. Phys. Solids 53(3), 655–679 (2005)
Scherge M., Gorb S.: Biological micro and nano tribilogy-Nature’s solutions. Springer, Berlin (2001)
Sha Y., Huy C.Y., Ruina A., Kramer E.J.: Detailed simulation of craze fibril failure at a crack tip in a glassy polymer. Acta Mat. 45(9), 3555–3563 (1997)
Theodorakopoulos N., Peyrard M., MacKay R.S.: Nonlinear structures and thermodynamic instabilities in a one-dimensional lattice system. Phys. Rev. Lett. 93, 258101 (2004)
Thomson, R.: Physics of fracture, vol. 39 of Solid State Physics. In: Turnbull, D., Ehrenreich, H. (eds.) pp. 1–129. Academic Press, New York (1987)
Yao H., Gao H.: Mechanics of robust and releasable adhesion in biology: bottom-up designed hierarchical structures of gecko. J. Mech. Phys. Solids 54, 1120–1146 (2006)
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by P. Suquet
Rights and permissions
About this article
Cite this article
Maddalena, F., Percivale, D., Puglisi, G. et al. Mechanics of reversible unzipping. Continuum Mech. Thermodyn. 21, 251–268 (2009). https://doi.org/10.1007/s00161-009-0108-2
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00161-009-0108-2