Event-Triggered $$H_{\infty }$$ Sliding Mode Control for Discrete-Time Singular Markov Jump Systems with Uncertainties in the Difference Matrix | Circuits, Systems, and Signal Processing Skip to main content
Springer Nature Link
Log in

Event-Triggered \(H_{\infty }\) Sliding Mode Control for Discrete-Time Singular Markov Jump Systems with Uncertainties in the Difference Matrix

  • Published:
Circuits, Systems, and Signal Processing Aims and scope Submit manuscript

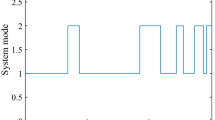
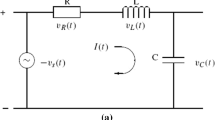
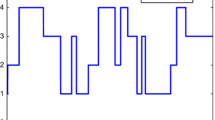
Abstract

This paper investigates the sliding mode control (SMC) problem of discrete-time singular Markovian jump systems with time-varying delay under an event-triggered strategy (ETS). In the system we study, there are uncertainties in the system matrix, especially in the difference matrix. A state augmentation strategy is proposed due to uncertainties in the difference matrix. A suitable sliding mode surface (SMS) function is built, and the corresponding sliding mode dynamics (SMD) is derived based on ETS. Some sufficient conditions are presented to guarantee that SMD is \(H_{\infty }\)-admissible for all uncertainties. Furthermore, an SMC law under ETS is derived so that the system trajectories can be driven onto the prescribed SMS in finite time. Finally, the effectiveness of the approach in the paper is demonstrated by two simulated examples.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

Data Availability

Data sharing is not applicable to this article as no datasets were generated or analyzed during the current study.

References

  1. T. Asai, S. Hara, Robust servo system design based on descriptor form representation with structured uncertainty. IFAC Proc. Vol. 26, 223–226 (1993)

    Article  Google Scholar 

  2. K.A. Barbosa, C.E. de Souza, D. Coutinho, Admissibility analysis of discrete linear time-varying descriptor systems. Automatica 91, 136–143 (2018)

    Article  MathSciNet  Google Scholar 

  3. X.H. Chang, L. Zhang, J.H. Park, Robust static output feedback \(H_{\infty }\) control for uncertain fuzzy systems. Fuzzy Sets Syst. 273, 87–104 (2015)

    Article  Google Scholar 

  4. J. Chen, S.Y. Xu, B.Y. Zhang, Z.D. Qi, Z. Li, Novel stability conditions for discrete-time T-S fuzzy systems: a Kronecker-product approach. Inf. Sci. 337, 72–81 (2016)

    Article  Google Scholar 

  5. L. Chen, X.M. Li, W.S. Lin, Q. Zhou, Adaptive event-triggered \(H_{\infty }\) control for Markov jump systems with generally uncertain transition rates. Circuits Syst. Signal Process. 39(11), 5429–5453 (2020)

    Article  Google Scholar 

  6. W.B. Chen, F. Gao, S.Y. Xu, Y.M. Li, Y.M. Chu, Robust stabilization for uncertain singular Markovian jump systems via dynamic output-feedback control. Syst. Control Lett. 171, 105433 (2023)

    Article  MathSciNet  Google Scholar 

  7. Z.G. Feng, Y. Yang, Robust \(H_{\infty }\) sliding mode control for uncertain polynomial fuzzy stochastic singular systems. J. Frankl. Inst. 360(4), 2893–2912 (2023)

    Article  MathSciNet  Google Scholar 

  8. Z. Gu, P. Shi, D. Yue, S. Yan, X.P. Xie, Memory-based continuous event-triggered control for networked T-S fuzzy systems against cyber attacks. IEEE Trans. Fuzzy Syst. 29(10), 3118–3129 (2021)

    Article  Google Scholar 

  9. Z. Lei, D.W.C. Ho, G.S. Zhai, Stability analysis of switched linear singular systems. Automatica 49(5), 1481–1487 (2013)

    Article  MathSciNet  Google Scholar 

  10. J.H. Li, Q.L. Zhang, X.G. Yan, S.K. Spurgeon, Observer-based fuzzy integral sliding mode control for nonlinear descriptor systems. IEEE Trans. Fuzzy Syst. 26(5), 2818–2832 (2018)

    Article  Google Scholar 

  11. C. Lin, Q.G. Wang, T.H. Lee, Robust normalization and stabilization of uncertain descriptor systems with norm-bounded perturbations. IEEE T. Autom. Control 50(4), 515–520 (2005)

    Article  MathSciNet  Google Scholar 

  12. Z.Y. Liu, C. Lin, B. Chen, Admissibility analysis for linear singular systems with time-varying delays via neutral system approach. ISA Trans. 61, 141–146 (2016)

    Article  Google Scholar 

  13. S.H. Long, L.J. Zhou, S.M. Zhong, D.X. Liao, An improved result for the finite-time stability of the singular system with time delay. J. Frankl. Inst. 359(16), 9006–9021 (2022)

    Article  MathSciNet  Google Scholar 

  14. D.G. Luenberger, Dynamic equations in descriptor form. IEEE Trans. Autom. Control 22(3), 312–321 (1977)

    Article  MathSciNet  Google Scholar 

  15. W.J. Mao, Robust stability and stabilisation of discrete-time descriptor systems with uncertainties in the difference matrix. IET Control Theory Appl. 6(17), 2676–2685 (2012)

    Article  MathSciNet  Google Scholar 

  16. Y.F. Mu, H.G. Zhang, S.X. Sun, J.C. Ren, Robust non-fragile proportional plus derivative state feedback control for a class of uncertain Takagi–Sugeno fuzzy singular systems. J. Frankl. Inst. 356(12), 6208–6225 (2019)

    Article  MathSciNet  Google Scholar 

  17. J.C. Ren, Q.L. Zhang, Robust \(H_{\infty }\) control for uncertain descriptor systems by proportional-derivative state feedback. Int. J. Control 83(1), 89–96 (2010)

    Article  MathSciNet  Google Scholar 

  18. J.C. Ren, Q.D. Sun, J. Fu, Robust sliding mode control for stochastic singular Markovian jump systems with unmatched one-sided Lipschitz nonlinearities. J. Frankl. Inst. 359(5), 1821–1851 (2022)

    Article  MathSciNet  Google Scholar 

  19. H. Shen, L. Su, J.H. Park, Extended passive filtering for discrete-time singular Markov jump systems with time-varying delays. Signal Process. 128, 68–77 (2016)

    Article  Google Scholar 

  20. F. Shen, P. Ju, M. Shahidehpour, Z.Y. Li, C. Wang, X.Y. Shi, Singular perturbation for the dynamic modeling of integrated energy systems. IEEE Trans. Power Syst. 35(3), 1718–1728 (2020)

    Article  Google Scholar 

  21. F. Shu, J.Y. Zhai, Observer-based global event-triggered control for a class of switched nonlinear systems. IEEE Trans. Syst. Man Cybern. Syst. 53(9), 5505–5515 (2023)

    Article  Google Scholar 

  22. X.J. Sun, Q.L. Zhang, Observer-based adaptive sliding mode control for T-S fuzzy singular systems. IEEE Trans. Syst. Man Cybern. Syst. 50(11), 4438–4446 (2020)

    Article  Google Scholar 

  23. Q.D. Sun, J.C. Ren, F. Zhao, Sliding mode control of discrete-time interval type-2 fuzzy Markov jump systems with the preview target signal. Appl. Math. Comput. 435, 127479 (2022)

    MathSciNet  Google Scholar 

  24. H.K. Sung, Delay-dependent stability analysis for singular Markovian jump systems with incomplete transition probabilities. J. Frankl. Inst. 352(1), 236–247 (2015)

    Article  MathSciNet  Google Scholar 

  25. J.H. Wang, Q.L. Zhang, X.X. Liu, \(H_{\infty }\) control for discrete-time singular Markovian jump systems based on the novel bounded real lemma. Int. J. Syst. Sci. 46(1), 63–75 (2015)

    Article  MathSciNet  Google Scholar 

  26. D.D. Wang, S.Z. Han, J. Chen, Robust admissibilization for discrete-time singular systems with time-varying delay. Math. Probl. Eng. 2019, 5368013 (2019)

    Article  MathSciNet  Google Scholar 

  27. Y. Wang, Y. Han, C. Gao, Robust \(H_{\infty }\) sliding mode control for uncertain discrete singular T-S fuzzy Markov jump systems. Asian J. Control 25(1), 524–536 (2023)

    Article  MathSciNet  Google Scholar 

  28. Z.Q. Wei, Y.C. Ma, Robust \(H_{\infty }\) observer-based sliding mode control for uncertain Takagi–Sugeno fuzzy descriptor systems with unmeasurable premise variables and time-varying delay. Inf. Sci. 566, 239–261 (2021)

    Article  MathSciNet  Google Scholar 

  29. L.G. Wu, Y.B. Gao, J.X. Liu, H.Y. Li, Event-triggered sliding mode control of stochastic systems via output feedback. Automatica 82, 79–92 (2017)

    Article  MathSciNet  Google Scholar 

  30. S. Xu, J. Lam, Robust Control and Filtering of Singular Systems (Springer, Berlin, 2006)

    Google Scholar 

  31. Q.Y. Xu, Y.J. Zhang, W.L. He, S.Y. Xiao, Event-triggered networked \(H_{\infty }\) control of discrete-time nonlinear singular systems. Appl. Math. Comput. 298, 368–382 (2017)

    MathSciNet  Google Scholar 

  32. D.Y. Yao, B. Zhang, P.S. Li, H.Y. Li, Event-triggered sliding mode control of discrete-time Markov jump systems. IEEE Trans. Syst. Man Cybern. Syst. 49(10), 2016–2025 (2019)

    Article  Google Scholar 

  33. M.M. Yuan, J.Y. Zhai, Dynamic event-triggered control for a class of p-normal nonlinear time-delay systems via output feedback. Int. J. Robust Nonlinear 33(1), 507–525 (2023)

    Article  MathSciNet  Google Scholar 

  34. Q.L. Zhang, J.H. Li, Z.Y. Song, Sliding mode control for discrete-time descriptor Markovian jump systems with two Markov chains. Optim. Lett. 12(6), 1199–1213 (2018)

    Article  MathSciNet  Google Scholar 

  35. D. Zhang, Y.W. Jing, Q.L. Zhang, G.M. Dimirovski, Stabilization of singular T-S fuzzy Markovian jump system with mode-dependent derivative-term coefficient via sliding mode control. Appl. Math. Comput. 364, 124643 (2020)

    MathSciNet  Google Scholar 

Download references

Acknowledgements

This work was supported by the National Natural Science Foundation of China under Grant 61673100 and the Fundamental Research Funds for the Central Universities under Grants N2224005-2, N232410019.

Author information

Authors and Affiliations

  1. College of Sciences, Northeastern University, Shenyang, 110819, People’s Republic of China

    Ying Su, Dongmei Yang & Junchao Ren

Authors
  1. Ying Su
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Dongmei Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Junchao Ren
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Dongmei Yang.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Su, Y., Yang, D. & Ren, J. Event-Triggered \(H_{\infty }\) Sliding Mode Control for Discrete-Time Singular Markov Jump Systems with Uncertainties in the Difference Matrix. Circuits Syst Signal Process 43, 4764–4789 (2024). https://doi.org/10.1007/s00034-024-02667-5

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00034-024-02667-5

Keywords

Search

Navigation