Electrothermal blood streaming conveying hybridized nanoparticles in a non-uniform endoscopic conduit

Abstract

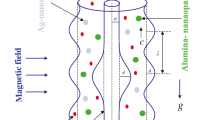
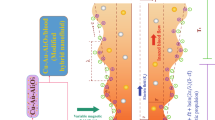
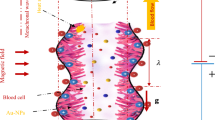
The novelty of nanoparticles in transferrals of medications and biological fluids via electrokinetic mechanism has been competently recognized. Due to the impressive role of nanoparticles suspended in blood or physiological fluids in medical fields, the current research article is planned to formulate an effective mathematical model to analyze the dynamism of bloodstream infused with hybridized nanoparticles in a non-uniform endoscopic conduit (space between two coaxial tubes) under the interactivities of electroosmosis, peristalsis, and buoyancy forces. The dual impact of heat source, Joule heating, and convectively cooling wall condition is examined. The geometrical shapes (sphere, brick, cylinder, and platelet) of nanoparticles injected into blood are accounted for in the formulation of modelled equations. The blood doped with hybridized nanoparticles is regarded as an electrolyte solution. The lubrication and Debye-Hückel linearization estimations are invoked in order to linearize the flow equations. Analytical solutions for the resulting leading equations are computed by implementing an analytical approach. The amendments in the physiognomies under variations in sundry parameters are explained through the line, bar graphs, and numerical tables. Outcomes admit that the flow of ionized blood is significantly amended across the endoscopic conduit due to the electrostatic body force. Blood is warmed or cooled with positive or negative values of Joule heating parameter. Blood is cooled with augmenting volumetric concentration of hybridized nanoparticles. The trapping phenomenon is also described by designing streamline plots. The size of confined blood boluses expands due to the thin electric double layer (EDL). The novel findings of this hemodynamic simulation furnish significant applicabilities in modelling of transportation of medications and drugs, physiological fluid mixers, testing and assessment of human diseases, detection of bacteria and viruses, etc.

Appendix

$$\begin{array}{@{}lll@{}} c_{1}&=& \frac{Y_{0}(-i \kappa r_{1})}{J_{0}(i \kappa r_{2}) Y_{0}(-i \kappa r_{1})-J_{0}(i \kappa r_{1}) Y_{0}(-i \kappa r_{2})},\\ c_{2}&=& \frac{J_{0}(i \kappa r_{1})}{J_{0}(i \kappa r_{1}) Y_{0}(-i \kappa r_{2})-J_{0}(i \kappa r_{2}) Y_{0}(-i \kappa r_{1})},\\ f_{1}&=&B_{i}-\frac{B_{i}}{4 x_{4}}(S x_{3}+\chi)({r_{2}^{2}}-{r_{1}^{2}})-\frac{1}{2} r_{2} (S x_{3}+\chi),\\ f_{2}&=&B_{i} \log{\frac{r_{2}}{r_{1}}}+\frac{x_{4}}{r_{2}},\\ c_{3}&=&-\frac{f_{1}}{f_{2}},\\ f_{3}&=&r_{2} B_{i} [{r_{2}^{2}} \log{r_{1}}(S x_{3}+\chi)-{r_{1}^{2}} \log {r_{2}} (S x_{3}+\chi)-4 x_{4} \log{r_{2}}]\\ &+&x_{4} [2 {r_{2}^{2}} \log {r_{1}}(S x_{3}+\chi)-{r_{1}^{2}} (S x_{3}+\chi)-4 x_{4}],\\ f_{4}&=&4 x_{4} [r_{2} B_{i} \log{\frac{r_{2}}{r_{1}}}+x_{4}],\\ c_{4}&=&-\frac{f_{3}}{f_{4}},\\ f_{5}&=& - Gr \kappa x_{2} [(S x_{3}+\chi)({r_{1}^{6}} -3 {r_{2}^{2}} {r_{1}^{4}}+3 {r_{2}^{4}} {r_{1}^{2}})+{r_{2}^{6}} \chi],\\ f_{6}&=& Gr \kappa {r_{2}^{6}} S x_{2} x_{3}+192 r_{1} U x_{4} [\kappa r_{1} \{c_{1} (I_{0}(\kappa r_{1}) +I_{0}(\kappa r_{2}))\\ &+&c_{2}\left(Y_{0}(-i \kappa r_{1})+Y_{0}(-i \kappa r_{2})\right)\}-4 i c_{2} Y_{1}(-i \kappa r_{1})],\\ f_{7}&=& -192 U_{hs} x_{4} [2 c_{1} \kappa {r_{1}^{2}} {{}_{0}}\tilde{F}_{1}\left(;2;\frac{1}{4} \kappa^{2} {r_{1}^{2}}\right) +r_{2} \{c_{1} \kappa r_{2} (I_{0}(\kappa r_{1})+I_{0}(\kappa r_{2})) -4 i c_{2} Y_{1}(-i \kappa r_{2})\}],\\ f_{8}&=& -192 \kappa x_{4} [(2 x_{1} ({r_{1}^{2}}-F)+{r_{2}^{2}} \{-2 x_{1}+U_{hs} (-2 c_{1} {{}_{0}}\tilde{F}_{1}\left(;2;\frac{1}{4} \kappa^{2} {r_{2}^{2}}\right)\\ &+&c_{2} Y_{0}(-i \kappa r_{1})+c_{2} Y_{0}(-i \kappa r_{2}))\}]-12 c_{3} Gr \kappa x_{2} x_{4} [-{r_{1}^{4}}+{r_{2}^{4}}+4 {r_{2}^{2}} {r_{1}^{2}}\log{\frac{r_{1}}{r_{2}}}],\\ f_{9}&=& 192 \kappa x_{1} x_{4} [{r_{1}^{2}} (\log{\frac{r_{1}}{r_{2}}}-1)+{r_{2}^{2}} (\log{\frac{r_{1}}{r_{2}}}+1)],\\ c_{5}&=&-\frac{1}{f_{9}}(f_{5}+f_{6}+f_{7}+f_{8}),\\ f_{10}&=& Gr \kappa \chi x_{2} [{r_{1}^{8}} \log{r_{2}}+{r_{2}^{2}} {r_{1}^{6}} (3-4 \log{r_{1}})+3 {r_{2}^{4}} {r_{1}^{4}} (\log{r_{1}r_{2}}-2)+{r_{2}^{6}} {r_{1}^{2}} (3-4 \log{r_{2}})+{r_{2}^{8}} \log{r_{1}}],\\ f_{11}&=& Gr \kappa {r_{1}^{2}} S x_{2} x_{3}[{r_{1}^{6}} \log{r_{2}}+{r_{2}^{2}} {r_{1}^{4}} (3-4 \log{r_{1}})+3 {r_{2}^{4}} {r_{1}^{2}}(\log{r_{1}r_{2}}-2)+{r_{2}^{6}}(3-4 \log{r_{2}})],\\ f_{12}&=& Gr \kappa {r_{2}^{8}} S x_{2} x_{3} \log{r_{1}}+192 {r_{1}^{3}} U_{hs} x_{4} [\kappa r_{1} \{c_{1} I_{0}(\kappa r_{2}) + c_{1}(\log{r_{1}}-1)\\ &+&c_{2} Y_{0}(-i \kappa r_{2})\}+4 i c_{2} \log{r_{2}} Y_{1}(-i \kappa r_{1})],\\ f_{13}&=& -192 \kappa {r_{1}^{4}} U_{hs} x_{4} [c_{1} \log{r_{1}} I_{0}(\kappa r_{2})+c_{1} \log{r_{2}}(I_{0}(\kappa r_{1})+1)+c_{2} \log{r_{1}} Y_{0}(-i \kappa r_{2})],\\ f_{14}&=& -192 {r_{1}^{2}} U_{hs} x_{4} \log{r_{2}} [\kappa {r_{1}^{2}} \{-2c_{1} {{}_{0}}\tilde{F}_{1}\left(;2;\frac{1}{4} \kappa^{2} {r_{1}^{2}}\right) +c_{2} Y_{0}(-i \kappa r_{1})\}+4 i c_{2} r_{2} Y_{1}(-i \kappa r_{2})],\\ f_{15}&=& -192 r_{1} {r_{2}^{2}} U_{hs} x_{4} [\kappa r_{1} \{c_{1} I_{0}(\kappa r_{1})+c_{1} I_{0}(\kappa r_{2})-2 c_{1}+c_{2} Y_{0}(-i \kappa r_{1})\} +4 i c_{2} \log{r_{1}} Y_{1}(-i \kappa r_{1})],\\ f_{16}&=& 192 \kappa {r_{1}^{2}} {r_{2}^{2}} U_{hs} x_{4}[2 \log{r_{1}} \{c_{1} I_{0}(\kappa r_{1})+c_{2} Y_{0}(-i \kappa r_{1})\}-c_{2} Y_{0}(-i \kappa r_{2})],\\ f_{17}&=&384 \kappa {r_{1}^{2}} {r_{2}^{2}} U_{hs} x_{4} [-c_{1} \log{r_{1}} {{}_{0}}\tilde{F}_{1}\left(;2;\frac{1}{4} \kappa^{2} {r_{1}^{2}}\right) +\log{r_{2}} \{(c_{1} I_{0}(\kappa r_{2})+c_{2} Y_{0}(-i \kappa r_{2})\}],\\ f_{18}&=&-192 {r_{2}^{2}} U_{hs} x_{4} [2 c_{1} \kappa {r_{1}^{2}} \log{r_{2}} {{}_{0}}\tilde{F}_{1}\left(;2;\frac{1}{4} \kappa^{2} {r_{2}^{2}}\right) +r_{2} \{c_{1} \kappa r_{2}-4 i c_{2} \log{r_{1}} Y_{1}(-i \kappa r_{2})\}], \end{array}$$

$$\begin{array}{@{}rcl@{}} f_{19}&=&192 \kappa {r_{2}^{4}} U_{hs} x_{4} [c_{1} I_{0}(\kappa r_{1})-c_{1} \log{r_{1}}(I_{0}(\kappa r_{2})+1)+c_{2} Y_{0}(-i \kappa r_{1})],\\ f_{20}&=&-192 \kappa {r_{2}^{4}} U_{hs} x_{4} [-2c_{1} \log{r_{1}} {{}_{0}}\tilde{F}_{1}\left(;2;\frac{1}{4} \kappa^{2} {r_{2}^{2}}\right) +c_{1} \log{r_{2}} (I_{0}(\kappa r_{1})-1)+c_{2} \log{r_{1}} Y_{0}(-i \kappa r_{2})],\\ f_{21}&=& -192 \kappa x_{4}[c_{2} {r_{2}^{4}} U_{hs} \log{r_{2}} Y_{0}(-i \kappa r_{1})-2 {r_{2}^{2}} x_{1} (F \log{r_{1}}+{r_{1}^{2}})\\ &+&{r_{1}^{2}} x_{1} \{2 F \log{r_{2}}-{r_{1}^{2}} (\log{r_{1}}+\log{r_{2}}-1)\}],\\ f_{22}&=&12 \kappa x_{4}[-c_{3} Gr {r_{1}^{6}} x_{2} \log{r_{2}}+c_{3} Gr {r_{2}^{2}} {r_{1}^{4}} x_{2} \log{r_{1}} (4 \log{r_{1}}-3)\\ &-&32 {r_{2}^{2}} {r_{1}^{2}} x_{1}\log{r_{1}r_{2}}+16 {r_{2}^{4}} x_{1} (\log{r_{1}r_{2}}-1)],\\ f_{23}&=& 12 c_{3} Gr \kappa {r_{1}^{2}} {r_{2}^{2}} x_{2} x_{4} [{r_{2}^{2}} (4 \log{r_{1}}-3 \log{r_{2}})-4 {r_{1}^{2}} (\log{r_{1}}-1) \log{r_{2}}],\\ f_{24}&=& -12 c_{3} Gr \kappa {r_{2}^{4}} x_{2} x_{4} [4 {r_{1}^{2}} \log{\frac{r_{1}}{r_{2}}} \log{r_{2}}+{r_{2}^{2}} \log{r_{1}}],\\ f_{25}&=&192 \kappa ({r_{1}^{2}}-{r_{2}^{2}}) x_{1} x_{4} [{r_{1}^{2}} (\log{\frac{r_{1}}{r_{2}}}-1)+{r_{2}^{2}}(\log{\frac{r_{1}}{r_{2}}}+1)],\\ c_{6}&=&-\frac{1}{f_{25}}(f_{10}+f_{11}+f_{12}+f_{13}+f_{14}+f_{15}+f_{16}+f_{17}+f_{18}\\ &+&f_{19}+f_{20}+f_{21}+f_{22}+f_{23}+f_{24}),\\ f_{26}&=&\frac{1}{8 x_{1}}({r_{1}^{4}}-{r_{2}^{4}}),\\ f_{27}&=&192 c_{1} \kappa r_{1} U_{hs} x_{4} [ {{}_{0}}\tilde{F}_{1}\left(;2;\frac{1}{4} \kappa^{2} {r_{1}^{2}}\right)-1] +384 i c_{2} U_{hs} x_{4} Y_{1}(-i \kappa r_{1})-Gr \kappa {r_{1}^{5}} x_{2} (S x_{3}+\chi),\\ f_{28}&=& 6 \kappa r_{1} x_{4} [Gr {r_{1}^{2}} x_{2} (4 c_{3} \log{r_{1}}-5c_{3}+4c_{4})-16 x_{1} (2c_{5} \log{r_{1}}-c_{5}+2c_{6})],\\ f_{29}&=& 192 c_{1} \kappa r_{2} U_{hs} x_{4} [ {{}_{0}}\tilde{F}_{1}\left(;2;\frac{1}{4} \kappa^{2} {r_{2}^{2}}\right)-1] +384 i c_{2} U_{hs} x_{4} Y_{1}(-i \kappa r_{2})-Gr \kappa {r_{2}^{5}} x_{2} (S x_{3}+\chi),\\ f_{30}&=& 6 \kappa r_{2} x_{4} [Gr {r_{2}^{2}} x_{2}(4 c_{3} \log{r_{2}}-5c_{3}+4c_{4})-16 x_{1}(2 c_{5} \log{r_{2}}-c_{5}+2 c_{6})],\\ f_{31}&=&192 \kappa x_{1} x_{4},\\ f_{32}&=&\frac{1}{384 \kappa x_{1} x_{4}},\\ f_{33}&=& 16 i c_{2} r_{2} U Y_{1}(-i \kappa r_{2})-\kappa {r_{2}^{4}}\left(\frac{dp}{dz}\right),\\ f_{34}&=& 4\kappa {r_{2}^{2}}[2 c_{1} U_{hs} \{ {{}_{0}}\tilde{F}_{1}\left(;2;\frac{1}{4} \kappa^{2} {r_{2}^{2}}\right)-1\}+x_{1} (-2 c_{5} \log{r_{2}}+c_{5}-2c_{6})],\\ f_{35}&=& Gr \kappa x_{2} [6 {r_{2}^{4}} x_{4}(4 c_{3} \log{r_{2}}-5c_{3}+4c_{4})-{r_{2}^{6}}(S x_{3}+\chi)] \end{array}$$