Design of three-dimensional dynamic integer tent map and its image encryption algorithm | Multimedia Tools and Applications Skip to main content
Springer Nature Link
Log in

Design of three-dimensional dynamic integer tent map and its image encryption algorithm

  • Published:
Multimedia Tools and Applications Aims and scope Submit manuscript

Abstract

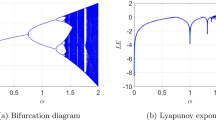
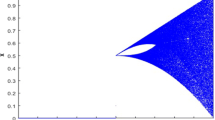
A three-dimensional dynamic integer tent map is proposed which is based on one-dimensional and two-dimensional integer tent maps, and the effects of dimension, accuracy and disturbance on the chaotic map system are studied. Experiments show that this system can quickly generate multiple integer pseudo-random sequences that are independent of each other and distributed uniformly, so that the problem of accuracy loss due to the integer real number model and the problem of insufficient independence and uniformity of a multi-dimensional model are solved. According to the characteristics of three-dimensional map systems, a color image encryption algorithm is designed and its performance is analyzed from different aspects.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11

Similar content being viewed by others

References

  1. Chen G, Mao Y, Chui CK (2004) A symmetric image encryption scheme based on 3D chaotic cat maps[J]. Chaos, Solitons and Fractals 21(3):749–761

    Article  MathSciNet  Google Scholar 

  2. Chen JX, Zhu ZL, Fu C, Yu H, Zhang LB (2015) An efficient image encryption scheme using gray code based permutation approach[J]. Opt Lasers Eng 67:191–204

    Article  Google Scholar 

  3. Chen F, Liu JD, Hu HH, Shang K (2019) Design and security simulation analysis of 2D integer tent mapping model [J]. Computer Engineering and Applications 55(01):103–108+173

    Google Scholar 

  4. Hermassi H, Belazi A, Rhouma R, Belghith SM (2013) Security analysis of an image encryption algorithm based on a DNA addition combining with chaotic maps[J]. Multimed Tools Appl 72(3):2211–2224

    Article  Google Scholar 

  5. Huang XL, Ye GD (2014) An image encryption algorithm based on hyper-chaos and DNA sequence[J]. Multimed Tools Appl 72(1):57–70

    Article  Google Scholar 

  6. Hussain I, Shah T, Gondal MA (2012) Image encryption algorithm based on PGL(2,GF(28)) S-boxes and TD-ERCS chaotic sequence[J]. Nonlinear Dynamics 70(1):181–187

    Article  MathSciNet  Google Scholar 

  7. Lei LH, Bai FM, Han XH (2013) New image encryption algorithm based on logistic map and hyper-Chaos[C]// International conference on computational and information sciences. IEEE.

    Google Scholar 

  8. Liu JD (2007) One-way hash function construction based on integer coupled tent maps[C]// International Symposium on Computer Science & Technology

    Google Scholar 

  9. Matthews R (1989) On the derivation of a "chaotic" encryption algorithm[J]. Cryptologia 13(1):29–41

    Article  MathSciNet  Google Scholar 

  10. Na SH, Jin SH, Kim SY, Ham BJ (2002) EEG in schizophrenic patients: mutual information analysis[J]. Clin Neurophysiol 113(12):1954–1960

    Article  Google Scholar 

  11. Pak C, Huang LL (2017) A new color image encryption using combination of the 1D chaotic map[J]. Signal Process 138:129–137

    Article  Google Scholar 

  12. Parvin Z, Seyedarabi H, Shamsi M (2016) A new secure and sensitive image encryption scheme based on new substitution with chaotic function[J]. Multimed Tools Appl 75(17):10631–10648

    Article  Google Scholar 

  13. Rukhin A, Soto J, Nechvatal J, Smid M, Barker R (2010) A statistical test suite for random and pseudorandom number generators for cryptographic applications[J]. Appl Phys Lett 22(7):1645–1179

    Google Scholar 

  14. Wang XY, Liu CM (2017) A novel and effective image encryption algorithm based on chaos and DNA encoding[J]. Multimed Tools Appl 76(5):6229–6245

    Article  Google Scholar 

  15. Wang W, Tan HY, Sun P, Pang Y, Ren BB (2016) A novel digital image encryption algorithm based on wavelet transform and multi-chaos[C]// 2015 2nd International Conference on Wireless Communication and Sensor Network (WCSN 2015).

    Google Scholar 

  16. Wang XY, Zhao YY, Zhang HL, Guo K (2016) A novel color image encryption scheme using alternate chaotic mapping structure. Optics & Lasers in Engineering 82:79–86

    Article  Google Scholar 

  17. Wang W, Si M, Pang Y et al (2017) An encryption algorithm based on combined chaos in body area networks[J]. Computers & Electrical Engineering:282–291

  18. Xu H, Deng Y (2018) Dependent evidence combination based on Shearman coefficient and Pearson coefficient[J]. IEEE Access 6:11634–11640

    Article  Google Scholar 

  19. Zhang YQ, Wang XY (2015) A new image encryption algorithm based on non-adjacent coupled map lattices[J]. Appl Soft Comput 26:10–20

    Article  Google Scholar 

  20. Zhang W, Wong KW, Yu H, Zhu ZL (2013) A symmetric color image encryption algorithm using the intrinsic features of bit distributions[J]. Commun Nonlinear Sci Numer Simul 18(3):584–600

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Information Engineering, Beijing Institute of Petrochemical Technology, Beijing, 102617, People’s Republic of China

    Liu Jiandong, Zhong Ming, Liu Bo, Liu Yujie & Li Bo

Authors
  1. Liu Jiandong
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Zhong Ming
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Liu Bo
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Liu Yujie
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Li Bo
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Liu Jiandong.

Additional information

Publisher’s note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Jiandong, L., Ming, Z., Bo, L. et al. Design of three-dimensional dynamic integer tent map and its image encryption algorithm. Multimed Tools Appl 80, 19219–19236 (2021). https://doi.org/10.1007/s11042-021-10668-8

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11042-021-10668-8

Keywords

Search

Navigation