Abstract
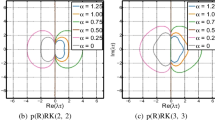
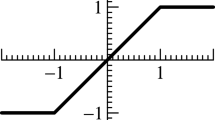
In this paper we give an analysis of the effect of stiff nonlinearities on the behavior of a Kaps-Rentrop method. To that end we introduce two quantities related to a simple model. The values of these quantities determine to some extent the behavior of a Kaps-Rentrop method in case of a strong coupling between the smooth component and the transient one. Numerical examples illustrate the theoretical results.
Zusammenfassung
In dieser Arbeit wird die Stabilität des Kaps-Rentrop-Verfahrens in die Anwesenheit nichtlinearer Steifheit (Stiffness) analysiert. Dazu werden mittels eines einfachen Modells zwei Größen introduziert. Die Werte dieser Größen reflektieren gewissermaßen das Verhalten eines Kaps-Rentrop-Verfahrens in die Anwesenheit einer bestimmten Kopplung zwischen die beiden Komponenten in das steife System gewöhnlicher Differentialgleichungen. Einige numerische Beispiele veranschaulichen die Analyse.
Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.References
Enright, W. H., Hull, T. E., Lindberg, B.: Comparing numerical methods for stiff systems of O.D.E.'s. BIT15, 10–48 (1975).
Gottwald, B. A., Wanner, G.: A reliable Rosenbrock integrator for stiff differential systems. Computing26, 335–360 (1981).
Kaps, P., Rentrop, P.: Generalized Runge-Kutta methods of order four with stepsize control for stiff ordinary differential equations. Numer. Math.33, 55–68 (1979).
Kaps, P., Wanner, G.: A study of Rosenbrock-type methods of high order. Numer. Math.38, 279–298 (1981).
Shampine, L. F.: Implementation of Rosenbrock methods. TOMS8, 93–113 (1982).
Stetter, H. J.: Towards a theory for discretizations of stiff differential systems. (Lecture Notes in Mathematics, Vol. 506, pp. 190–210.) Berlin-Heidelberg-New York: Springer 1976.
Veldhuizen, M. van: D-stability. SINUM18, 45–64 (1981).
Verwer, J. G.: Instructive experiments with some Runge-Kutta-Rosenbrock methods. Comp. & Math. with Appls.8, 217–229 (1982).
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
van Veldhuizen, M. D-stability and Kaps-Rentrop-methods. Computing 32, 229–237 (1984). https://doi.org/10.1007/BF02243574
Received:
Issue Date:
DOI: https://doi.org/10.1007/BF02243574