Abstract
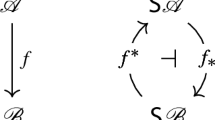
We establish a 2-categorical duality involving the 2-category A κ of all κ-accessible categories with wide pullbacks, also known as locally κ-polypresentable categories, and of functors preserving κ-filtered colimits and wide pullbacks. Commutation of wide pullbacks with so-called quasi-coproducts in Set is the basic ingredient to this duality, which leads to a full characterization of categories of type Wdpb Filtκ (A, Set)=A κ
Similar content being viewed by others
References
Adámek, J. and Rosický, J.: Accessible Categories and Locally Presentable Categories, Cambridge University Press, 1994.
Adámek, J. and Volger, H.: On locally reflective categories of structures, Preprint, Universität Passau, 1992.
Ageron, P.: The logic of structures, J. Pure appl. Algebra 79 (1992), 15–34.
Artin, M., Grothendieck, A., and Verdier, J.L., Théorie des Topos et Cohomologie Etale des 021 Schémas, Lecture Notes in Math. 269 and 270, Springer, Berlin, 1972.
Barr, M.: Representations of categories, J. Pure Appl. Algebra 41 (1986), 113–137.
Börger, R. and Tholen, W.: Abschwächungen des Adjunktionsbegriffs, Manuscripta Math. 19 (1976), 19–45.
Börger, R. and Tholen, W.: Strong, regular and dense generators, Cahiers Top. Géom. Diff. Cat. 32 (1990), 257–276.
Carboni, A. and Johnstone, P. T.: Connected limits, familial representability and Artin glueing, Preprint, Cambridge University, 1993.
Coste, M.: Localisations dans les catégories de modéles, Thèse, Université Paris Nord, 1977.
Diers, Y.: Familles universelles de morphismes, Ann. Soc. Bruxelles 93 (1979), 175–195.
Diers, Y.: Catégories localement multiprésentables, Arch. Math. 34 (1980), 344–356.
Girard, J. Y.: The system F of variable types fifteen years later, Theoret. Comput. Sci. 45 (1986), 159–192.
Guitart, R. and Lair, C.: Existence de diagrammes localement libres, I, Diagram 6 (1981), GL1-GL13.
Gabriel, P. and Ulmer, F.: Lokal präsentierbare Kategorien, Lecture Notes in Math. 221, 021 Springer, Berlin, 1971.
Hébert, M.: Syntactic characterization of closure underconnected limits, Arch. Math. Logic 31 (1991), 133–143.
Hébert, M.: Syntactic characterizations of closure under pullbacks and of locally polypresentable categories, Preprint, Université Laval, 1993.
Hu, H.: Duality for accessible categories, in Can. Math. Soc. Conference Proceedings, Vol. 13, A.M.S., Providence, 1992, pp. 211–242.
Hu, H. and Tholen, W.: Limits in free coproduct completions, J. Pure Appl. Algebra, to appear.
Johnstone, P. T.: A syntactic approach to Diers' localizable categories, in Applications of Sheaves, Lecture Notes in Math. 753, Springer, Berlin, 1979, pp. 466–478.
Kaput, J. J.: Locally adjunctable functors, Ill. J. Math. 16 (1972), 86–94.
Kelly, G. M.: Basic Concepts of Enriched Category Theory, Cambridge University Press, 1982.
Lair, C.: Catégories modelables et catégories esquissables, Diagrammes 7 (1981), L1-L20.
Lamarche, F.: Modelling polymorphism with categories, PhD thesis, McGill University, 1988.
Mac Lane, S.: Categories for the Working Mathematician, Springer, Berlin, 1971.
Makkai, M.: Strong conceptual completeness for first order logic, Ann. Pure Appl. Logic 40 (1988), 167–215.
Makkai, M.: A theorem on Barr-exact categories, with an infinitary generalization, Ann. Pure Appl. Logic 47 (1990), 225–268.
Makkai, M. and Paré, R.: Accessible categories: the foundations of categorical model theory, Contemporary Math. 104, A.M.S., Providence, 1990.
Makkai, M. and Pitts, A.: Some results on locally finitely presentable categories, Trans. Amer. Math. Soc. 229 (1987), 473–496.
Paré, R.: Simply connected limits, Can J. Math. 42 (1990), 731–746.
Taylor, P.: The trace factorization of stable functors, Preprint, Imperial College, 1990.
Taylor, P.: Locally finitely poly-presentable categories, Preprint, Imperial College, 1990.
Tholen, W.: MacNeille completion of concrete categories with local properties, Comment. Math. Univ. St. Pauli 28 (1979), 179–202.
Volger, H.: Preservation theorems for limits of structures and global sections of sheaves of structures, Math. Z. 166 (1979), 27–53.
Author information
Authors and Affiliations
Additional information
The first author acknowledges financial assistance from a special research grant of the Faculty of Arts at York University. The second author is partially supported by an NSERC operating grant.
Rights and permissions
About this article
Cite this article
Hu, H., Tholen, W. Quasi-coproducts and accessible categories with wide pullbacks. Appl Categor Struct 4, 387–402 (1996). https://doi.org/10.1007/BF00122686
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.1007/BF00122686