Two-Step Estimation for Modeling the Earthquake Occurrences in Sumatra by Neyman–Scott Cox Point Processes | SpringerLink
Skip to main content
Springer Nature Link
Log in

Two-Step Estimation for Modeling the Earthquake Occurrences in Sumatra by Neyman–Scott Cox Point Processes

  • Conference paper
  • First Online:
Soft Computing in Data Science (SCDS 2021)

Part of the book series: Communications in Computer and Information Science ((CCIS,volume 1489))

Included in the following conference series:

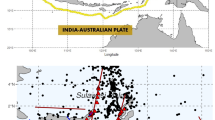
Abstract

The Cox point process is highly considered for earthquake modeling. However, the complex earthquake data which involve a large number of occurrences and geological variables often require expensive computation. This study aims to propose an efficient algorithm based on the two-step procedure by constructing the first and second order composite likelihoods. We consider four Neyman–Scott Cox process models and apply them to fit the earthquake distribution in Sumatra. We conclude that the Cauchy cluster process performs best.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Chapter
JPY 3498
Price includes VAT (Japan)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever
eBook
JPY 10295
Price includes VAT (Japan)
  • Available as EPUB and PDF
  • Read on any device
  • Instant download
  • Own it forever
Softcover Book
JPY 12869
Price includes VAT (Japan)
  • Compact, lightweight edition
  • Dispatched in 3 to 5 business days
  • Free shipping worldwide - see info

Tax calculation will be finalised at checkout

Purchases are for personal use only

Institutional subscriptions

Similar content being viewed by others

References

  1. Ogata, Y.: Statistical models for earthquake occurrences and residual analysis for point processes. J. Am. Stat. Assoc. 83(401), 9–27 (1988)

    Article  Google Scholar 

  2. Zhuang, J., Ogata, Y., Vere-Jones, D.: Stochastic declustering of space-time earthquake occurrences. J. Am. Stat. Assoc. 97(458), 369–380 (2002)

    Article  MathSciNet  Google Scholar 

  3. Türkyilmaz, K., van Lieshout, M.N.M., Stein, A.: Comparing the Hawkes and trigger process models for aftershock sequences following the 2005 Kashmir earthquake. Math. Geosci. 45(2), 149–164 (2013)

    Article  Google Scholar 

  4. Choiruddin, A., Aisah, Trisnisa, F., Iriawan, N.: Quantifying the effect of geological factors on distribution of earthquake occurrences by inhomogeneous Cox processes. Pure Appl. Geophys. 178(5), 1579–1592 (2021)

    Google Scholar 

  5. Aisah, I.N., Choiruddin, A.: On the earthquake modeling by using Bayesian mixture Poisson process. Int. J. Adv. Sci. Technol. 29(7s), 3350–3358 (2020)

    Google Scholar 

  6. Mukhti, T.O., Choiruddin, A., Purhadi: Generalized additive Poisson models for quantifying geological factors effect on the earthquake risk mapping. J. Phys. Conf. Ser. 1863(1), p. 012030 (2021)

    Google Scholar 

  7. Siino, M., Adelfio, G., Mateu, J., Chiodi, M., D’Alessandro, A.: Spatial pattern analysis using hybrid models: an application to the Hellenic seismicity. Stoch. Environ. Res. Risk Assess 31(7), 1633–1648 (2016). https://doi.org/10.1007/s00477-016-1294-7

    Article  Google Scholar 

  8. Møller, J., Waagepetersen, R.P.: Statistical Inference and Simulation for Spatial Point Processes. CRC Press (2003)

    Google Scholar 

  9. Møller, J., Waagepetersen, R.P.: Modern statistics for spatial point processes. Scand. J. Stat. 34(4), 643–684 (2007)

    MathSciNet  MATH  Google Scholar 

  10. Waagepetersen, R.P., Guan, Y.: Two-step estimation for inhomogeneous spatial point processes. J. Roy. Stat. Soc.: Ser. B (Stat. Methodol.) 71(3), 685–702 (2009)

    Article  MathSciNet  Google Scholar 

  11. Guan, Y., Shen, Y.: A weighted estimating equation approach for inhomogeneous spatial point processes. Biometrika 97(4), 867–880 (2010)

    Article  MathSciNet  Google Scholar 

  12. Guan, Y.: A composite likelihood approach in fitting spatial point process models. J. Am. Stat. Assoc. 101(476), 1502–1512 (2006)

    Article  MathSciNet  Google Scholar 

  13. Natawidjaja, D.H.: Tectonic setting indonesia dan pemodelan sumber gempa dan tsunami. Geoteknologi-LIPI (2007)

    Google Scholar 

  14. Amri, M.R., et al.: Risiko bencana Indonesia. Badan Nasional Penanggulangan Bencana, Jakarta (2016)

    Google Scholar 

  15. Baddeley, A., Rubak, E., Turner, R.: Spatial Point Patterns: Methodology and Applications with R. CRC Press (2015)

    Google Scholar 

  16. Choiruddin, A., Coeurjolly, J.-F., Letué, F., et al.: Convex and non-convex regularization methods for spatial point processes intensity estimation. Electron. J. Stat. 12(1), 1210–1255 (2018)

    Article  MathSciNet  Google Scholar 

  17. Choiruddin, A., Coeurjolly, J.-F., Waagepetersen, R.P.: Information criteria for inhomogeneous spatial point processes. Aust. New Zealand J. Stat. 63(1), 119–143 (2021)

    Article  MathSciNet  Google Scholar 

  18. Jalilian, A., Guan, Y., Waagepetersen, R.P.: Decomposition of variance for spatial Cox processes. Scand. J. Stat. 40(1), 119–137 (2013)

    Article  MathSciNet  Google Scholar 

  19. Choiruddin, A., Coeurjolly, J.-F., Letué, F.: Adaptive lasso and Dantzig selector for spatial point processes intensity estimation. arXiv preprint arXiv:2101.03698 (2021)

Download references

Acknowledgements

The research is supported by Institut Teknologi Sepuluh Nopember grant number 1292/PKS/ITS/2021. We thank the two reviewers for the comments.

Author information

Authors and Affiliations

  1. Department of Statistics, Institut Teknologi Sepuluh Nopember (ITS), Surabaya, Indonesia

    Achmad Choiruddin, Tabita Yuni Susanto & Rahma Metrikasari

Authors
  1. Achmad Choiruddin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Tabita Yuni Susanto
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Rahma Metrikasari
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Achmad Choiruddin .

Editor information

Editors and Affiliations

  1. Universiti Teknologi MARA, Shah Alam, Malaysia

    Azlinah Mohamed

  2. Universiti Teknologi MARA, Shah Alam, Selangor, Malaysia

    Bee Wah Yap

  3. Universiti Teknologi MARA, Shah Alam, Malaysia

    Jasni Mohamad Zain

  4. University of Tennessee, Knoxville, TN, USA

    Michael W. Berry

Rights and permissions

Reprints and permissions

Copyright information

© 2021 Springer Nature Singapore Pte Ltd.

About this paper

Check for updates. Verify currency and authenticity via CrossMark

Cite this paper

Choiruddin, A., Susanto, T.Y., Metrikasari, R. (2021). Two-Step Estimation for Modeling the Earthquake Occurrences in Sumatra by Neyman–Scott Cox Point Processes. In: Mohamed, A., Yap, B.W., Zain, J.M., Berry, M.W. (eds) Soft Computing in Data Science. SCDS 2021. Communications in Computer and Information Science, vol 1489. Springer, Singapore. https://doi.org/10.1007/978-981-16-7334-4_11

Download citation

  • DOI: https://doi.org/10.1007/978-981-16-7334-4_11

  • Published:

  • Publisher Name: Springer, Singapore

  • Print ISBN: 978-981-16-7333-7

  • Online ISBN: 978-981-16-7334-4

  • eBook Packages: Computer ScienceComputer Science (R0)

Publish with us

Policies and ethics

Search

Navigation