Dynamical Analysis of a Novel Chaotic Circuit | SpringerLink
Skip to main content
Springer Nature Link
Log in

Dynamical Analysis of a Novel Chaotic Circuit

  • Conference paper
  • First Online:
Bio-inspired Computing: Theories and Applications (BIC-TA 2017)

Part of the book series: Communications in Computer and Information Science ((CCIS,volume 791))

  • 1132 Accesses

Abstract

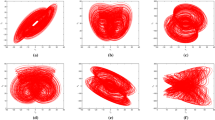
Chaotic circuit is an effective tool to observe and analyze chaotic phenomena, to verify chaos theory and to promote its application. The recent research work focuses on how to better analyze the basic circuit characteristics and to design application circuits. In this paper, a new chaotic system is proposed, whose dynamical behaviors are discussed with the change of the parameters in detail. The specific effects of different parameters on the system are also discussed. By adjusting these parameters of the proposed circuit, this nonlinear circuit can produce the different dynamical behaviors, such as, hyper chaotic behavior, periodic behavior, transient behavior, etc. In addition, the simulation results of Matlab can further prove the feasibility of this circuit.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Chapter
JPY 3498
Price includes VAT (Japan)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever
eBook
JPY 5719
Price includes VAT (Japan)
  • Available as EPUB and PDF
  • Read on any device
  • Instant download
  • Own it forever
Softcover Book
JPY 7149
Price includes VAT (Japan)
  • Compact, lightweight edition
  • Dispatched in 3 to 5 business days
  • Free shipping worldwide - see info

Tax calculation will be finalised at checkout

Purchases are for personal use only

Institutional subscriptions

Similar content being viewed by others

References

  1. Lü, J.G.: Chaotic dynamics of the fractional-order Lü system and its synchronization. Phys. Lett. A 354, 483–489 (2006)

    Google Scholar 

  2. Lü, J.H., Chen, G.R., Cheng, D.Z.: A new chaotic system and beyond: the generalized Lorenz-like system. Int. J. Bifurc. Chaos 14, 1507–1537 (2004)

    Article  MATH  MathSciNet  Google Scholar 

  3. Lü, J.G.: Generating chaos via decentralized linear state feedback and a class of nonlinear functions. Chaos Solitons Frac. 25, 403–413 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  4. Feki, M.: Observer-based synchronization of chaotic systems with unknown nonlinear function. Chaos Solitons Frac. 39, 981–990 (2009)

    Article  MathSciNet  Google Scholar 

  5. Wan, Z.C.: Synchronization between two different chaotic systems using nonlinear control function. Microelectr. Comput. 25, 52–55 (2008)

    Google Scholar 

  6. Chua, L.O., Kang, S.M.: Memristive device and systems. IEEE Proc. 64, 209–223 (1976)

    Article  MathSciNet  Google Scholar 

  7. Lorenz, E.N.: Deterministic nonperiodic flow. J. Atmosph. Sci. 20, 130–141 (1963)

    Article  Google Scholar 

  8. Li, Q., Hu, S., Tang, S., Zeng, G.: Hyperchaos and horseshoe in a 4D memristive system with a line of equilibria and its implementation. Int. J. Circ. Theor. Appl. 42, 1172–1188 (2013)

    Article  Google Scholar 

  9. Iu, H.H.C., Yu, D.S., Fitch, A.L., Sreeram, V., Chen, H.: Controlling chaos in a memristor based circuit using a twint notch filter. IEEE Trans. Circ. Syst. 58, 1337–1344 (2011)

    Article  Google Scholar 

  10. Bao, B.C., Ma, Z.H., Xu, J.P., Liu, Z., Xu, Q.: A simple memristor chaotic circuit with complex dynamics. Int. J. Bifurc. Chaos 21, 2629–2645 (2011)

    Article  MATH  Google Scholar 

  11. Buscarino, A., Fortuna, L., Frasca, M., Gambuzza, L.: A chaotic circuit based on Hewlett-Packard memristor. Chaos 22, 23–36 (2012)

    MATH  MathSciNet  Google Scholar 

  12. Diaz, G., Coimbra, C.F.M.: Nonlinear dynamics and control of a variable order oscillator with application to the Van der Pol equation. Nonlinear Dyn. 56, 145–157 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  13. Vincent, U.E., Nbendjo, B.R.N., Ajayi, A.A., Njah, A.N., Mcclintock, P.V.E.: Hyperchaos and bifurcations in a driven Van der Pol-duffing oscillator circuit. Int. J. Dyn. Control 3, 363–370 (2015)

    Article  MathSciNet  Google Scholar 

  14. Matouk, A.E.: Chaos, feedback control and synchronization of a fractional-order modified autonomous Van der Pol-Duffing circuit. Chaos Soliton Fract 16, 975–986 (2011)

    MATH  MathSciNet  Google Scholar 

  15. Jia, Q.: Hyperchaos generated from the Lorenz chaotic system and its control. Phys. Lett. A 336, 217–222 (2007)

    Article  MATH  Google Scholar 

  16. Rössler, O.E.: An equation for continuous chaos. Phys. Lett. A 57, 397–398 (1976)

    Article  MATH  Google Scholar 

  17. Li, C., Chen, G.: Chaos in the fractional order Chen system and its control. Commun. Nonlinear Sci. 22, 549–554 (2004)

    MATH  Google Scholar 

  18. Bi, Q.S., Ma, R., Zhang, Z.D.: Bifurcation mechanism of the bursting oscillations in periodically excited dynamical system with two time scales. Nonlinear Dyn. 79, 101–110 (2015)

    Article  MathSciNet  Google Scholar 

  19. Radwan, A.G., Soliman, A.M., El-Sedeek, A.L.: An inductorless CMOS realization of Chua’s circuit. Chaos Solitons Frac. 18, 149–158 (2003)

    Article  Google Scholar 

  20. Radwan, A.G., Soliman, A.M., El-Sedeek, A.L.: MOS realization of the conjectured simplest chaotic equation. Circ. Syst. Signal Proc. 22, 277–285 (2003)

    MATH  MathSciNet  Google Scholar 

  21. Bao, B.C., Liu, Z., Xu, J.P.: Steady periodic memristor oscillator with transient chaotic behaviors. Electr. Lett. 46, 237–238 (2010)

    Google Scholar 

  22. Bao, B.C., Ma, Z.H., Xu, J.P., Liu, Z., Xu, Q.: A simple memristor chaotic circuit with complex dynamics. Int. J. Bifurc. Chaos 21, 2629–2645 (2011)

    Article  MATH  Google Scholar 

  23. Fitch, A.L., Yu, D., Iu, H., Sreeram, V.: Hyperchaos in a memristor-based modified canonical Chua’s circuit. Int. J. Bifurc. Chaos 22, 125–133 (2015)

    MATH  Google Scholar 

  24. Liu, H., Kadir, A., Li, Y.: Asymmetric color pathological image encryption scheme based on complex hyper chaotic system. Int. J. Light Electr. Optics 127, 5812–5819 (2016)

    Article  Google Scholar 

  25. Tokida, C., Saito, T.: On a synchronization phenomena in third order autonomous chaotic circuit. Am. J. Phys. 72, 379–385 (2012)

    Google Scholar 

  26. Kilic, R., Saracoglu, O.G., Yildirim, F.: Experimental observations of EMI effects in autonomous Chuas chaotic circuit. Chaos Solitons Frac. 32, 1168–1177 (2007)

    Article  Google Scholar 

  27. Koliopanos, C.L., Kyprianidis, I.M., Stouboulos, I.N., Anagnostopoulos, A.N., Magafas, L.: Chaotic behaviour of a fourth-order autonomous electric circuit. Chaos Solitons Fract. 16, 173–182 (2003)

    Article  MATH  Google Scholar 

  28. Sun, J.W., Wu, Y.Y., Cui, G.Z.: Finite-time Real combination synchronization of three complex-variable chaotic systems with unknown parameters via sliding mode control. Nonlinear Dyn. 88, 1677–1690 (2017)

    Article  Google Scholar 

  29. Sun, J.W., Wang, Y., Wang, Y.F., Shen, Y.: Finite-time synchronization between two complex-variable chaotic systems with unknown parameters via nonsingular tterminal sliding mode control. Nonlinear Dyn. 85, 1105–1117 (2016)

    Article  MATH  Google Scholar 

  30. Sun, J.W., Shen, Y.: Quasi-ideal Memory System. IEEE Trans. Cyber. 45, 1353–1362 (2015)

    Article  Google Scholar 

Download references

Acknowledgments

The work is supported by the State Key Program of National Natural Science of China (Grant No. 61632002), the National Natural Science of China (Grant Nos. 61572446, 61472372, 61603347, 61603348, 61602424), Science and Technology Innovation Talents Henan Province (Grant No. 174200510012), Research Program of Henan Province (Grant Nos. 15IRTSTHN012, 162300410220, 17A120005), and the Science Foundation of for Doctorate Research of Zhengzhou University of Light Industry (Grant No. 2014BSJJ044).

Author information

Authors and Affiliations

  1. Henan Key Lab of Information-Based Electrical Appliances, Zhengzhou University of Light Industry, Zhengzhou, 450002, China

    Junwei Sun, Nan Li & Yanfeng Wang

  2. College of Electrical and Electronic Engineering, Zhengzhou University of Light Industry, Zhengzhou, 450002, China

    Junwei Sun, Nan Li & Yanfeng Wang

Authors
  1. Junwei Sun
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Nan Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Yanfeng Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yanfeng Wang .

Editor information

Editors and Affiliations

  1. School of Automation, Huazhong University of Science and Technology, Wuhan, China

    Cheng He

  2. Automation College, Harbin Engineering University, Harbin, China

    Hongwei Mo

  3. School of Automation, Huazhong University of Science and Technology, Wuhan, China

    Linqiang Pan

  4. Automation College, Harbin Engineering University, Harbin, China

    Yuxin Zhao

Rights and permissions

Reprints and permissions

Copyright information

© 2017 Springer Nature Singapore Pte Ltd.

About this paper

Check for updates. Verify currency and authenticity via CrossMark

Cite this paper

Sun, J., Li, N., Wang, Y. (2017). Dynamical Analysis of a Novel Chaotic Circuit. In: He, C., Mo, H., Pan, L., Zhao, Y. (eds) Bio-inspired Computing: Theories and Applications. BIC-TA 2017. Communications in Computer and Information Science, vol 791. Springer, Singapore. https://doi.org/10.1007/978-981-10-7179-9_27

Download citation

  • DOI: https://doi.org/10.1007/978-981-10-7179-9_27

  • Published:

  • Publisher Name: Springer, Singapore

  • Print ISBN: 978-981-10-7178-2

  • Online ISBN: 978-981-10-7179-9

  • eBook Packages: Computer ScienceComputer Science (R0)

Publish with us

Policies and ethics

Search

Navigation