Abstract
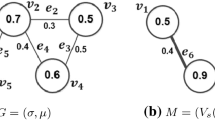
We present a new graph matching approach based on 1D information. Each node of the graphs represents a fuzzy region (fuzzy segmentation step). Each couple of nodes is linked by a relational histogram which can be assumed to the attraction of two regions following a set of directions. The attraction is computed by a continuous function, depending on the distance of the confronted objects. Each case of the histogram corresponds to a particular direction. Then, relational graph computed from strong scenes are matched.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Preview
Unable to display preview. Download preview PDF.
Similar content being viewed by others
References
Barni, M., Cappellini, V., Mecocci, A.: Comments on “A Possibilistic Approach to Clustering”. IEEE Trans. Fuzzy Systems 4, 393–396 (1996).
Berztiss, A. T.: A backtrack procedure for isomorphism of directed graphs. Assoc. Comput. Mach. 20, 365–377 (1973).
Bezdek, J. C.: Pattern recognition with fuzzy objective function algorithms. New York: Plenum Press, 1981.
Dave, R. N.: Characterization and detection of noise in clustering. Pattern Rec. Lett. 12, 657–664 (1991).
Dubois, D., Jaulent, M. C.: A general approach to parameter evaluation in fuzzy digital pictures. Pattern Rec. Lett. 6, 251–261 (1987).
Dhérété, P., Wendling, L., Desachy, J.: Fuzzy segmentation and astronomical images interpretation. ICLAP’95-IAPR, Lecture Notes in Computer Science, vol. 974, pp 21–27. Berlin Heidelberg New York Tokyo: Springer 1995.
Jantzen, J., Ring, P.: Image segmentation based on scaled membership functions. 2nd IEEE Conference on Fuzzy Systems 2, pp. 714–718 (1993).
Krishnapuram, R., Keller, J. M., Ma, Y.: Quantitative analysis of properties and spatial relations of fuzzy images regions, IEEE Trans. Fuzzy System 1, 222–233 (1993).
Krishnapuram, R.: Generation of membership functions via possibilistic clustering. 3rd IEEE Conf. Fuzzy System 3, 902–908 (1994).
Keller, J. M., Carpenter, C. L.: Image segmentation in the presence of uncertainty. Int. J. Intell. Syst. 5, 193–208 (1990).
Krishnapuram, R.: Fuzzy clustering methods in computer vision. EUFIT’93, pp. 720–730 (1993).
Krishnapuram, R., Keller, J. M.: A possibilistic approach to clustering. IEEE Trans Fuzzy Syst. 1, 98–110(1993).
Krishnapuram, R., Keller, J. M.: The possibilistic C-means algorithm: insights and recommendations. IEEE Trans. Fuzzy Systems 4, 385–393 (1996).
Horowitz, S. L., Pavlidis, T.: Picture segmentation by a directed split and merge procedure. 2nd International Conference on Pattern Recognition, pp. 424–433 (1974).
Matsakis, P., Wendling, L., Desachy, J.: Représentation de la position relative d’objets 2D au moyen d’un histogramme de forces. Rev. Trait. Signal 4, 63–76 (1998).
Miyajima, K., Ralescu, A.: Spatial organization in 2D segmented images: representation and recognition of primitive spatial relations. Fuzzy Sets Systems 65, 225–236 (1994).
Rosenfeld, A.: Fuzzy geometry: an overview. First IEEE Conf. Fuzzy Systems, pp. 113–118 (1992).
Rosenfeld, A., Hummel, R., Zucker, S.: Scene labelling by relaxation operations. IEEE Trans. Syst. Man Cybernetics 6, 420–433 (1976).
Ruspini, E. H.: A new approach to clustering. Inf. Control 15, 22–32 (1969).
Wendling, L.: Segmentation floue appliquée à la reconnaissance d’objets dans les images numériques. Ph.D. thesis, Université Paul Sabatier, Toulouse, 1997.
Wendling, L., Pariès, A., Desachy, J.: Pattern recognition by splitting images into trees of fuzzy regions. Int. J. Intell. Data Anal. 1(2) (1997). http://www-east.elsevier.com/ida
H. J. Zimmerman, P. Zysno, Quantifying vagueness in decision models. Eur. J. Oper. Res. 22, 148–158 (1985).
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 1998 Springer-Verlag Wien
About this paper
Cite this paper
Wendling, L., Desachy, J. (1998). Isomorphism between Strong Fuzzy Relational Graphs Based on k-Formulae. In: Jolion, JM., Kropatsch, W.G. (eds) Graph Based Representations in Pattern Recognition. Computing Supplement, vol 12. Springer, Vienna. https://doi.org/10.1007/978-3-7091-6487-7_7
Download citation
DOI: https://doi.org/10.1007/978-3-7091-6487-7_7
Publisher Name: Springer, Vienna
Print ISBN: 978-3-211-83121-2
Online ISBN: 978-3-7091-6487-7
eBook Packages: Springer Book Archive