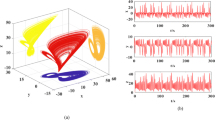
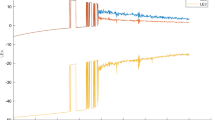
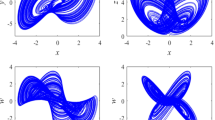
Abstract
It is shown how a four-wing hyper-chaotic attractor can be used for secure communication using parameter convergence. Using some variables for complete replacement and some for feedback control and unknown parameter adaptation, two hyper-chaotic attractors are synchronized in a time less than the time scale of their chaotic oscillations. This synchronization is used for and secure communication of digital messages. The coding parameter of the transmitting system changes so rapidly that an intruder cannot infer any information about the attractors corresponding to the two coding parameters. The scheme presented in this paper is more secure as compared to other similar schemes. This is demonstrated by comparison with an existing scheme based on parameter adaptation and Lyapunov stability theory.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Preview
Unable to display preview. Download preview PDF.
Similar content being viewed by others
References
Pecora, L.M., Carroll, T.L.: Synchronization in chaotic systems. Phys. Rev. Lett. 64, 821–825 (1990)
Pecora, L.M., Carroll, T.L.: Driving systems with chaotic signals. Phys. Rev. A. 44, 2374–2384 (1991)
Cuomo, K.M., Oppenheim, A.V.: Circuit implementation of synchronized chaos with applications to communications. Phys. Rev. Lett. 71, 65–68 (1993)
Kocarev, L., Parlitz, U.: General approach for chaotic synchronization with applications to communication. Phys. Rev. Lett. 74, 5028–5031 (1995)
Kennedy, M.P., Kolumban, G.: Digital communication using chaos. Signal Processing 80, 1307–1320 (2000)
Dedieu, H., Kennedy, M.P., Hasler, M.: Chaos Shift Keying: Modulation and Demodulation of chaotic carrier using self-synchronizing Chua’s circuit. IEEE Transaction on Circuit and System-II Analog and Digital Signal Processing 40, 634–642 (1993)
Pecora, L.M., Carroll, T.L., Johnson, G.A., Mar, D.J., Heagy, J.F.: Fundamentals of synchronization in chaotic systems, concepts and applications. Chaos 7, 520–543 (1997)
Dwivedi, A., Mittal, A.K., Dwivedi, S.: Adaptive synchronization of diffusionless Lorenz systems and secure communication of digital signals by parameter modulation. IET Communications 6, 2016–2026 (2012)
Feki, M.: An adaptive chaos synchronization scheme applied to secure communication. Chaos, Solitons and Fractals 18, 141–148 (2003)
Adloo, H., Roopaei, M.: Review article on adaptive synchronization of chaotic systems with unknown parameters. Nonlinear Dyn. 65, 141–159 (2011)
Chen, S., Lu, J.: Synchronization of uncertain unified chaotic system via adaptive control. Chaos, Solitons and Fractals 14, 643–647 (2002)
Dong, E., Chen, Z., Yuan, Z., Chen, Z.: A New Four-wing Hyper-chaotic Attractor and Its Circuit Implementation. In: International Workshop on Chaos-Fractal Theory and its Applications (2010)
Perez, G., Cerdeira, H.A.: Extracting messages masked by chaos. Phys. Rev. Lett. 74, 1970–1973 (1995)
Jovic, B., Unsworth, C.P., Berber, S.M.: De-noising Initial Condition Modulation Wideband Chaotic Communication Systems with Linear & Wavelet Filters. In: Proceedings of the First IEEE International Conference on Wireless Broadband and Ultra Wideband Communications (Aus Wireless 2006), Sydney, Australia, pp. 1–6 (2006)
Grzesiak, M.: Wavelet filtering of chaotic data. Nonlinear Processes in Geophysics 7, 111–116 (2000)
Broomhead, D., Huke, J., Muldoon, M.: Linear Filters and Nonlinear Systems. Journal of the Royal Statistical Society 54, 373–382 (1992)
Roy, M., Kumar, V., Kulkarni, B., Sanderson, J., Rhodes, M., Stappen, M.: Simple denoising algorithm using wavelet transform. AIChE Journal 45, 2461–2466 (1999)
Constantine, W., Reinhall, P.: Wavelet-based in-band denoising technique for chaotic sequences. International Journal of Bifurcation and Chaos 11, 483–495 (2000)
Boccalleti, S., Guiaquinta, A., Arecchi, F.: Adaptive recognition and filtering of noise using wavelets. Physical Review E 55, 5393–5397 (1997)
Carroll, T.L.: Approximating chaotic time series through unstable periodic orbits. Physical Review E 59, 1615–1621 (1999)
Alvarez, G., Montoya, F., Romera, M., Pastor, G.: Breaking parameter modulated chaotic secure communication system. Chaos, Solitons and Fractals 21, 783–787 (2004)
Yin, P., Min, L., Li, P.: A text encryption scheme based generalized discrete chaos synchronization for image communication. In: International Conference on Environmental Engineering and Technology. Advances in Biomedical Engineering, vol. 8, pp. 310–316 (2012)
Yau, H.-T., Pu, Y.-C., Li, S.-C.: Application of chaotic synchronization system to secure communication. Information Technology and Control 41, 274–282 (2012)
Huang, C.K., Liao, C.W., Hsu, S.L., Jeng, Y.C.: Implementation of gray image encryption with pixel shuffling and gray-level encryption by single chaotic system. Telecommunication Syst., 1–9 (2011)
Sengodan, V., Balamurugan, A.: Efficient signal encryption using chaos based system. International Journal of Electronics Engineering 2, 335–338 (2010)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2014 Springer-Verlag Berlin Heidelberg
About this paper
Cite this paper
Dwivedi, A., Mittal, A.K., Dwivedi, S. (2014). Secure Communication Using Four-Wing Hyper-Chaotic Attractor. In: Mauri, J.L., Thampi, S.M., Rawat, D.B., Jin, D. (eds) Security in Computing and Communications. SSCC 2014. Communications in Computer and Information Science, vol 467. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-662-44966-0_27
Download citation
DOI: https://doi.org/10.1007/978-3-662-44966-0_27
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-662-44965-3
Online ISBN: 978-3-662-44966-0
eBook Packages: Computer ScienceComputer Science (R0)