Faster Way to Agony

Abstract

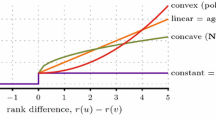
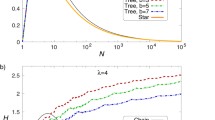
Many real-world phenomena exhibit strong hierarchical structure. Consequently, in many real-world directed social networks vertices do not play equal role. Instead, vertices form a hierarchy such that the edges appear mainly from upper levels to lower levels. Discovering hierarchies from such graphs is a challenging problem that has gained attention. Formally, given a directed graph, we want to partition vertices into levels such that ideally there are only edges from upper levels to lower levels. From computational point of view, the ideal case is when the underlying directed graph is acyclic. In such case, we can partition the vertices into a hierarchy such that there are only edges from upper levels to lower edges. In practice, graphs are rarely acyclic, hence we need to penalize the edges that violate the hierarchy. One practical approach is agony, where each violating edge is penalized based on the severity of the violation. The fastest algorithm for computing agony requires O(nm ²) time. In the paper we present an algorithm for computing agony that has better theoretical bound, namely O(m ²). We also show that in practice the obtained bound is pessimistic and that we can use our algorithm to compute agony for large datasets. Moreover, our algorithm can be used as any-time algorithm.