Abstract
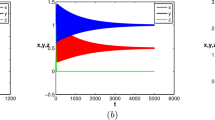
The principle aim of this paper is to explore the existence of periodic solution of a predator-prey model with stage structure for the predator and impulsive perturbations. Sufficient and realistic conditions are obtained by using Mawhin’s continuation theorem of the coincidence degree. Further, some numerical simulations show that our model can occur in many forms of complexities including periodic oscillation and chaotic strange attractor.
This is a preview of subscription content, log in via an institution to check access.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Preview
Unable to display preview. Download preview PDF.
Similar content being viewed by others
References
Georgesc, P., Hsien, Y.H.: Global dynamics of a predator-prey model with stage structure for the predator. Society for Industrial and Applied Mathematics 67, 1379–1395 (2007)
Chen, L.J., Chen, F.D.: A stage-structure and harvesting predator-prey system. Annals of Differential Equations 03, 293–301 (2011)
Zhao, M., Cheng, R.F.: Positive Periodic Solution in a Ratio-Based Predator-Prey System with Stage Structure for Predator. Journal of Biomathematics 25, 88–96 (2010)
Lai, W.Y., Zhan, Q.Y.: Permanence and Global Stability of a Predator-prey System with Stage Structure and Time Delays. College Mathematics 28, 50–57 (2012)
Ma, Z.H., Wang, S.F., Wang, W.T., Li, Z.Z.: Permanence of a stage-structured predator-prey system with a class of functional responses. Comptes Rendus Biologies 334, 851–854 (2011)
Zhang, Z.Q., Wang, Z.C.: Periodic Solutions of a Predator-Prey System with Stage-Structure for Predator. Acta Mathematica Sinica 48, 541–548 (2005)
Ling, L.S.: Stability and Hopf Bifurcation in a Predator-prey Model with Stage Structure and Time Delay. Mathematica Applicata 25, 131–139 (2012)
Wang, W.: Global dynamics of a population model with stage structure for predator. In: Advanced Topics in Biomathematics, pp. 253–257. World Scientific, River Edge (1997)
Wang, W., Chen, L.: A predator-prey system with stage structure for predator. Comput. Math. Appl. 33, 83–91 (1997)
Gaines, R.E., Mawhin, J.L.: Coincidence degree and nonlinear differential equations. Springer, Berlin (1977)
Zhang, J., Gui, J.: Periodic solutions of nonautonomous cellular neural networks with impulses and delays. Nonlinear Analysis: Real World Applications 19, 1891–1903 (2009)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2012 Springer-Verlag Berlin Heidelberg
About this paper
Cite this paper
Wang, K., Zhang, W., Gui, Z. (2012). Existence and Simulations of Periodic Solution for Impulsive Predator-Prey System with Stage Structure for the Predator. In: Liu, B., Ma, M., Chang, J. (eds) Information Computing and Applications. ICICA 2012. Lecture Notes in Computer Science, vol 7473. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-642-34062-8_15
Download citation
DOI: https://doi.org/10.1007/978-3-642-34062-8_15
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-642-34061-1
Online ISBN: 978-3-642-34062-8
eBook Packages: Computer ScienceComputer Science (R0)