Abstract
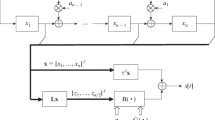
A method of computing the number of 1′ s and 0′ s as well as the number of runs of any length in the sequence obtained from a LFSR-based generator has been developed. The procedure that is based on the decomposition of the generating function in global minterms allows one to check balancedness and run distribution goodness in the output sequence. Simple design rules are also derived.
This is a preview of subscription content, log in via an institution to check access.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Preview
Unable to display preview. Download preview PDF.
Similar content being viewed by others
References
Golomb, S.W.: Shift Register-Sequences. Aegean Park Press, Laguna Hill (1982)
Key, E.L.: An Analysis of the Structure and Complexity of Non-Linear Binary Sequence Generators. IEEE Trans. on Information Theory 22, 732–736 (1976)
Klapper, A.: Large Families of Sequences with Near Optimal Correlations and Large Linear Span. IEEE Trans. on Information Theory 42, 1241–1248 (1996)
Kumar, P.V., Scholtz, R.A.: Bounds on the Linear Span of Bent Sequences. IEEE Trans. on Information Theory 29, 854–862 (1983)
Lidl, R., Niederreiter, H.: Introduction to Finite Fields and Their Applications. Cambridge University Press, Cambridge (1986)
MacWilliams, F.J., Sloane, N.J.: The Theory of Error-Correcting Codes. North-Holland Mathematical Library. Elsevier Science Publishers, Amsterdam (1977)
Mange, D.: Analysis and Synthesis of Logic Systems. Artech House, INC., Norwood (1986)
Meier, W., Staffelbach, O.: Nonlinearity Criteria for Cryptographic Functions. In: Quisquater, J.-J., Vandewalle, J. (eds.) EUROCRYPT 1989. LNCS, vol. 434, pp. 549–563. Springer, Heidelberg (1990)
Menezes, A.J., et al.: Handbook of Applied Cryptography. CRC Press, New York (1997)
Rueppel, R.A.: Analysis and Design of Stream Ciphers. Springer, New York (1986)
Shparlinski, I.E.: On the Linear Complexity of the Power Generator. Design, Codes and Cryptography 23, 5–10 (2001)
Simmons, G.J. (ed.): Contemporary Cryptology: The Science of Information Integrity. IEEE Press, New York (1991)
Tilborg, H.C.: An Introduction to Cryptology. Kluwer Academic Publishers, Norwell (1988)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2003 Springer-Verlag Berlin Heidelberg
About this paper
Cite this paper
Fúster-Sabater, A. (2003). Aspects of Pseudorandomness in Nonlinear Generators of Binary Sequences. In: Blundo, C., Laneve, C. (eds) Theoretical Computer Science. ICTCS 2003. Lecture Notes in Computer Science, vol 2841. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-540-45208-9_26
Download citation
DOI: https://doi.org/10.1007/978-3-540-45208-9_26
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-540-20216-5
Online ISBN: 978-3-540-45208-9
eBook Packages: Springer Book Archive