Positivity and Invariance Properties of Nonisothermal Tubular Reactor Nonlinear Models

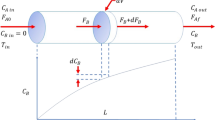
Abstract

The existence and uniqueness of the state trajectories (temperature and reactant concentration) and the existence and multiplicity of equilibrium profiles are analyzed for a nonisothermal axial dispersion tubular reactor model. It is reported that the trajectories exist on the whole (nonnegative real) time axis and the set of all physically feasible state values is invariant under the dynamics equations. The main nonlinearity in the model originates from the Arrhenius-type kinetics term in the model equations. The analysis uses Lipschitz and dissipativity properties of the nonlinear operator involved in the dynamics and the concept of state trajectory positivity. In addition, the multiplicity of the equilibrium profiles is reported: there is at least one steady state among the physically feasible states for such models, and conditions which ensure the multiplicity of equilibrium profiles are given.