Abstract
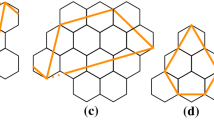
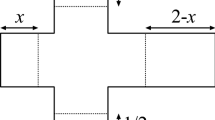
The structure of the set of all convex polyhedra foldable from a square is detailed. It is proved that five combinatorially distinct nondegenerate polyhedra, and four different flat polyhedra, are realizable. All the polyhedra are continuously deformable into each other, with the space of polyhedra having the topology of four connected rings.
This is a preview of subscription content, log in via an institution to check access.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Preview
Unable to display preview. Download preview PDF.
Similar content being viewed by others
References
Aleksandrov, A.D.: Konvexe Polyeder. Akademie Verlag, Berlin (1958)
Lubiw, A., O’Rourke, J.: When can a polygon fold to a polytope? Technical Report 048, Dept. Comput. Sci., Smith College (1996) Presented at AMS Conf., October 5 (1996)
Demaine, E.D., Demaine, M.L., Lubiw, A., O’Rourke, J.: Examples, counterexamples, and enumeration results for foldings and unfoldings between polygons and polytopes. Technical Report 069, Smith College, Northampton, MA, LANL ArXive cs.CG/0007019 (2000)
Demaine, E.D., Demaine, M.L., Lubiw, A., O’Rourke, J.: Enumerating foldings and unfoldings between polygons and polytopes. Graphs and Combinatorics 18, 93–104 (2002)
O’Rourke, J.: Folding and unfolding in computational geometry. In: Akiyama, J., Kano, M., Urabe, M. (eds.) JCDCG 1998. LNCS, vol. 1763, pp. 258–266. Springer, Heidelberg (2000), Papers from the Japan Conf. Discrete Comput. Geom., Tokyo (December 1998)
Shephard, G.C.: Convex polytopes with convex nets. Math. Proc. Camb. Phil. Soc. 78, 389–403 (1975)
Aleksandrov, A.D.: Existence of a convex polyhedron and a convex surface with a given metric. In: Reshetnyak, Y.G., Kutateladze, S.S. (eds.) A. D. Aleksandrov: Selected Works: Part I, Gordon and Breach, Australia, pp. 169–173 (1996); Translation of Doklady Akad. Nauk SSSR, Matematika 30(2), 103–106 (1941)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2003 Springer-Verlag Berlin Heidelberg
About this paper
Cite this paper
Alexander, R., Dyson, H., O’Rourke, J. (2003). The Foldings of a Square to Convex Polyhedra. In: Akiyama, J., Kano, M. (eds) Discrete and Computational Geometry. JCDCG 2002. Lecture Notes in Computer Science, vol 2866. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-540-44400-8_5
Download citation
DOI: https://doi.org/10.1007/978-3-540-44400-8_5
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-540-20776-4
Online ISBN: 978-3-540-44400-8
eBook Packages: Springer Book Archive