Abstract
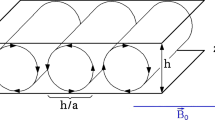
The flow of an electrolyte in a shallow square horizontal cavity subject to a steady current between opposite sides in the presence of an array of external magnets is simulated using a two dimensional lattice Boltzmann equation method for different values of the Chandrasekhar number. The flow is in a viscous regime for small values of Ch and in an advective one for larger values. In this last regime and in a steady state, a fixed number of pairs of initially close ideal tracer particles are added to the flow. We find that the average distance between each pair grows exponentially in time. Then, an average Lyapunov exponent that grows as a power law of the Chandrasekhar number can be defined.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Similar content being viewed by others
References
Tabeling, P., Cardoso, O., Perrin, B.: Chaos in a linear array of vortices. J. Fluid Mech. 213, 511–530 (1990)
Paret, J., et al.: Are flows electromagnetically forced in thin stratified layers two dimensional? Phys. Fluids 9(10), 3102–3104 (1997). https://doi.org/10.1063/1.869419
Rothstein, D., Henry, E., Gollub, J.P.: Persistent patterns in transient chaotic fluid mixing. Nature 401(6755), 770–772 (1999). https://doi.org/10.1038/44529
Oulette, N.T., Gollub, J.P.: Curvature fields, topology, and the dynamics of spatiotemporal chaos. Phys. Rev. Lett. 99, 194502 (2007)
Rechtman, R., et al.: Competition of localized thermal buoyancy and Lorentz forces in an electrolyte enclosed in a cavity. Phys. Fluids 33, 127115 (2021)
Succi, S.: The Lattice Boltzmann Equation. Numerical Mathematics and Scientific Computation. Clarendon Press, Oxford (2001)
Wolf-Gladrow, D.A.: Lattice-Gas Cellular Automata and Lattice Boltzmann Models. An Introduction. LNM, vol. 1725, pp. 1–13. Springer, Heidelberg (2000)
Bhatnagar, P.L., Gross, E.P., Krook, M.: A model for collision processes in gases. I. Small amplitude processes in charged and neutral one-component systems. Phys. Rev. 94(3), 511– 525 (1954). https://doi.org/10.1103/physrev.94.511
Inamuro, T., et al.: A lattice Boltzmann method for a binary miscible fluid mixture and its application to a heat-transfer problem. J. Comput. Phys. 179, 201–215 (2002)
Shan, X.: Simulation of Rayleigh–Bénard convection using a lattice Boltzmann method. Phys. Rev. E 55(3), 2780–2788 (1997)
He, X., Chen, S., Doolen, G.D.: A novel thermal model for the lattice Boltzmann method in incompressible limit. J. Comp. Phys. 146, 282–300 (1998)
Buick, J.M., Greated, C.A.: Lattice Boltzmann modeling of interfacial gravity waves. Phys. Fluids 10, 1490–1511 (1998)
Lallemand, P., Luo, L.S.: Theory of the lattice Boltzmann method: acoustic and thermal properties in two and three dimensions. Phys. Rev. E 68, 036706 (2003)
Chen, S., Doolen, G.D.: Lattice Boltzmann method for fluid flows. Annu. Rev. Fluid Mech. 30(1), 329–364 (1998). https://doi.org/10.1146/annurev.fluid.30.1.329
McCaig, M.: Permanent Magnets in Theory and Practice. Wiley, Hoboken (1952)
Cuevas, S., Smolentsev, S., Abdou, M.A.: On the flow past a magnetic obstacle. J. Fluid Mech. 553, 227–252 (2006)
Román, J., Figueroa, A., Cuevas, S.: Wake patterns behind a magnetic obstacle in an electrolyte layer. Magnetohydrodynamics 53(1), 55–66 (2017)
Duran-Matute, M., Trieling, R.R., Van Heijst, G.J.F.: Scaling and asymmetry in an electromagnetically forced dipolar flow structure. Phys. Rev. E 83(1), 016306 (2011)
Wolf, A.: 13. Quantifying chaos with Lyapunov exponents. In: Chaos, pp. 273–290. Princeton University Press (1986). https://doi.org/10.1515/9781400858156.273
Fouxon, I., et al.: Reynolds number dependence of Lyapunov exponents of turbulence and fluid particles. Phys. Rev. E 103(3), 033110 (2021). https://doi.org/10.1103/physreve.103.033110
Artale, V., et al.: Dispersion of passive tracers in closed basins: beyond the diffusion coefficient. Phys. Fluids 9, 3162–3171 (1997)
Boffetta, G., et al.: Experimental evidence of chaotic advection in a convective flow. Europhys. Lett. 48(6), 629 (1999). https://doi.org/10.1209/epl/i1999-00530-3
Bian, F., Shi, D., Sun, L., Bao, F.: An efficient approach for computing the finite time Lyapunov exponent in complex three-dimensional flow based on the discrete phase model. Aerosp. Sci. Technol. 133, 108110 (2023)
Author information
Authors and Affiliations
Corresponding author
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2024 The Author(s), under exclusive license to Springer Nature Switzerland AG
About this paper
Cite this paper
Bagnoli, F., Rechtman, R. (2024). Chaos in a Two-Dimensional Magneto-Hydrodynamic System. In: Bagnoli, F., Baetens, J., Bandini, S., Matteuzzi, T. (eds) Cellular Automata. ACRI 2024. Lecture Notes in Computer Science, vol 14978. Springer, Cham. https://doi.org/10.1007/978-3-031-71552-5_9
Download citation
DOI: https://doi.org/10.1007/978-3-031-71552-5_9
Published:
Publisher Name: Springer, Cham
Print ISBN: 978-3-031-71551-8
Online ISBN: 978-3-031-71552-5
eBook Packages: Computer ScienceComputer Science (R0)