Abstract
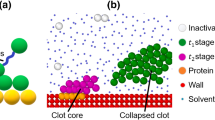
The stability of the initial platelet aggregates is relevant in both hemostasis and thrombosis. Understanding the structural stresses of such aggregates under different flow conditions is crucial to gaining insight into the role of platelet activation and binding in the more complex process of clot formation. In this work, a three-dimensional implicit partitioned fluid-structure interaction (FSI) model is presented to study the deformation and structural stress of platelet aggregates in specific blood flow environments. Platelet aggregates are considered as porous mediums in the model. The FSI model couples a fluid solver based on Navier-Stokes equations and a porosity-dependent compressible neo-Hookean material to capture the mechanical characteristics of the platelet aggregates. A parametric study is performed to explore the influence of porosity and applied body force on this material. Based on in vitro experimental data, the deformation and associated stress of a low shear aggregate and a high shear aggregate under different flow conditions are evaluated. This FSI framework offers a way to elucidate the complex interaction between blood flow and platelet aggregates and is applicable to a wider range of porous biomaterials in flow.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Similar content being viewed by others
References
Barsimantov, J., et al.: Poroelastic characterization and modeling of subcutaneous tissue under confined compression. Ann. Biomed. Eng. (2024)
Bath, P., Butterworth, R.: Platelet size: measurement, physiology and vascular disease. Blood Coagul. Fibrinol. Int. J. Haemostasis Thrombosis 7(2), 157–161 (1996)
Bershadsky, E.S., Ermokhin, D.A., Kurattsev, V.A., Panteleev, M.A., Nechipurenko, D.Y.: Force balance ratio is a robust predictor of arterial thrombus stability. Biophys. J. (2024)
Blom, F.J.: A monolithical fluid-structure interaction algorithm applied to the piston problem. Comput. Methods Appl. Mech. Eng. 167(3), 369–391 (1998)
Boodt, N., et al.: Mechanical characterization of thrombi retrieved with endovascular thrombectomy in patients with acute ischemic stroke. Stroke 52(8), 2510–2517 (2021)
Cahalane, R.M.E., et al.: Tensile and compressive mechanical behaviour of human blood clot analogues. Ann. Biomed. Eng. 51(8), 1759–1768 (2023)
van Dam, E.A., et al.: Non-linear viscoelastic behavior of abdominal aortic aneurysm thrombus. Biomech. Model. Mechanobiol. 7(2), 127–137 (2008)
Degroote, J.: Partitioned simulation of fluid-structure interaction. Arch. Comput. Methods Eng. 20(3), 185–238 (2013)
Degroote, J., Haelterman, R., Annerel, S., Vierendeels, J.: Coupling techniques for partitioned fluid-structure interaction simulations with black-box solvers. In: MpCCI User Forum, 10th, Proceedings, pp. 82–91. Fraunhofer Institute SCAI (2009)
Felippa, C.A., Park, K., Farhat, C.: Partitioned analysis of coupled mechanical systems. Comput. Methods Appl. Mech. Eng. 190(24), 3247–3270 (2001). Adv. Comput. Methods Fluid-Struct. Interact
Fernández, M.A.: Coupling schemes for incompressible fluid-structure interaction: implicit, semi-implicit and explicit. SeMA J. 55(1), 59–108 (2011)
Han, D., Zhang, J., He, G., Griffith, B.P., Wu, Z.J.: A prestressed intracellular biomechanical model for the platelet to capture the disc-to-sphere morphological change from resting to activated state. Int. J. Comput. Methods 19(10), 2250021 (2022)
Hao, Y., Závodszky, G., Tersteeg, C., Barzegari, M., Hoekstra, A.G.: Image-based flow simulation of platelet aggregates under different shear rates. PLOS Comput. Biol. 19(7), 1–20 (2023)
Hecht, F.: New development in FreeFem++. J. Numer. Math. 20(3–4), 251–265 (2012)
Huang, C.C., Shih, C.C., Liu, T.Y., Lee, P.Y.: Assessing the viscoelastic properties of thrombus using a solid-sphere-based instantaneous force approach. Ultrasound Med. Biol. 37(10), 1722–1733 (2011)
Kadri, O.E., Chandran, V.D., Surblyte, M., Voronov, R.S.: In vivo measurement of blood clot mechanics from computational fluid dynamics based on intravital microscopy images. Comput. Biol. Med. 106, 1–11 (2019)
Küttler, U., Wall, W.A.: Fixed-point fluid-structure interaction solvers with dynamic relaxation. Comput. Mech. 43(1), 61–72 (2008)
Lee, S., Jang, S., Park, Y.: Measuring three-dimensional dynamics of platelet activation using 3-d quantitative phase imaging. bioRxiv (2019)
Maas, S.A., Ellis, B.J., Ateshian, G.A., Weiss, J.A.: FEBio: finite elements for biomechanics. J. Biomech. Eng. 134(1), 011005 (2012)
Matthies, H.G., Niekamp, R., Steindorf, J.: Algorithms for strong coupling procedures. Comput. Methods Appl. Mech. Eng. 195(17), 2028–2049 (2006)
Rugonyi, S., Bathe, K.: On the analysis of fully-coupled fluid flows with structural interactions - a coupling and condensation procedure. Int. J. Comput. Civil Struct. Eng. 1, 29–41 (2000)
Slaboch, C.L., Alber, M.S., Rosen, E.D., Ovaert, T.C.: Mechano-rheological properties of the murine thrombus determined via nanoindentation and finite element modeling. J. Mech. Behav. Biomed. Mater. 10, 75–86 (2012)
Stalker, T.J., et al.: Hierarchical organization in the hemostatic response and its relationship to the platelet-signaling network. Blood 121(10), 1875–1885 (2013)
Storti, F., van de Vosse, F.N.: A continuum model for platelet plug formation, growth and deformation. Int. J. Numer. Methods Biomed. Eng. 30(12), 1541–1557 (2014)
Teeraratkul, C., Tomaiuolo, M., Stalker, T.J., Mukherjee, D.: Investigating clot-flow interactions by integrating intravital imaging with in silico modeling for analysis of flow, transport, and hemodynamic forces. Sci. Rep. 14(1), 696 (2024)
Trudnowski, R.J., Rico, R.C.: Specific gravity of blood and plasma at 4 and \(37^{\circ }\text{C}\). Clin. Chem. 20(5), 615–616 (1974)
Voronov, R.S., Stalker, T.J., Brass, L.F., Diamond, S.L.: Simulation of intrathrombus fluid and solute transport using in vivo clot structures with single platelet resolution. Ann. Biomed. Eng. 41(6), 1297–1307 (2013)
Wang, W., Lindsey, J.P., Chen, J., Diacovo, T.G., King, M.R.: Analysis of early thrombus dynamics in a humanized mouse laser injury model. Biorheology 51(3–14), 1 (2014)
Welsh, J.D., et al.: A systems approach to hemostasis: 1. The interdependence of thrombus architecture and agonist movements in the gaps between platelets. Blood 124(11), 1808–1815 (2014)
Winterstein, A., Lerch, C., Bletzinger, K.U., Wüchner, R.: Partitioned simulation strategies for fluid-structure-control interaction problems by Gauss-Seidel formulations. Adv. Model. Simul. Eng. Sci. 5(1), 29 (2018)
Author information
Authors and Affiliations
Corresponding author
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2024 The Author(s), under exclusive license to Springer Nature Switzerland AG
About this paper
Cite this paper
Hao, Y., Hoekstra, A.G., Závodszky, G. (2024). A Three-Dimensional Fluid-Structure Interaction Model for Platelet Aggregates Based on Porosity-Dependent Neo-Hookean Material. In: Franco, L., de Mulatier, C., Paszynski, M., Krzhizhanovskaya, V.V., Dongarra, J.J., Sloot, P.M.A. (eds) Computational Science – ICCS 2024. ICCS 2024. Lecture Notes in Computer Science, vol 14838. Springer, Cham. https://doi.org/10.1007/978-3-031-63783-4_5
Download citation
DOI: https://doi.org/10.1007/978-3-031-63783-4_5
Published:
Publisher Name: Springer, Cham
Print ISBN: 978-3-031-63785-8
Online ISBN: 978-3-031-63783-4
eBook Packages: Computer ScienceComputer Science (R0)