Abstract
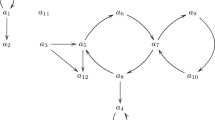
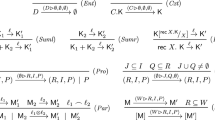
In the area of Natural Computing, reaction systems are a qualitative abstraction inspired by the functioning of living cells, suitable to model the main mechanisms of biochemical reactions. This model has already been applied and extended successfully to various areas of research. Reaction systems interact with the environment represented by the context, and pose problems of implementation, as it is a new computation model. In this paper we consider the link-calculus, which allows to model multiparty interaction in concurrent systems, and show that it allows to embed reaction systems, by representing the behaviour of each entity and preserving faithfully their features. We show the correctness and completeness of our embedding. We illustrate our framework by showing how to embed a lac operon regulatory network. Finally, our framework can contribute to increase the expressiveness of reaction systems, by exploiting the interaction among different reaction systems.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Similar content being viewed by others
Notes
- 1.
After ‘chained Core Network Algebra’.
References
Azimi, S.: Steady states of constrained reaction systems. Theor. Comput. Sci. 701(C), 20–26 (2017). https://doi.org/10.1016/j.tcs.2017.03.047
Azimi, S., Iancu, B., Petre, I.: Reaction system models for the heat shock response. Fundam. Inf. 131(3–4), 299–312 (2014). https://doi.org/10.3233/FI-2014-1016
Barbuti, R., Gori, R., Levi, F., Milazzo, P.: Investigating dynamic causalities in reaction systems. Theor. Comput. Sci. 623, 114–145 (2016)
Bernini, A., Brodo, L., Degano, P., Falaschi, M., Hermith, D.: Process calculi for biological processes. Nat. Comput. 17(2), 345–373 (2018)
Bodei, C., Brodo, L., Bruni, R.: Open multiparty interaction. In: Martí-Oliet, N., Palomino, M. (eds.) WADT 2012. LNCS, vol. 7841, pp. 1–23. Springer, Heidelberg (2013). https://doi.org/10.1007/978-3-642-37635-1_1
Bodei, C., Brodo, L., Bruni, R., Chiarugi, D.: A flat process calculus for nested membrane interactions. Sci. Ann. Comp. Sci. 24(1), 91–136 (2014)
Bodei, C., Brodo, L., Gori, R., Levi, F., Bernini, A., Hermith, D.: A static analysis for Brane Calculi providing global occurrence counting information. Theoret. Comput. Sci. 696, 11–51 (2017)
Bodei, C., Brodo, L., Bruni, R.: A formal approach to open multiparty interactions. Theoret. Comput. Sci. 763, 38–65 (2019)
Brijder, R., Ehrenfeucht, A., Main, M., Rozenberg, G.: A tour of reaction systems. Int. J. Found. Comput. Sci. 22(07), 1499–1517 (2011)
Brodo, L.: On the expressiveness of pi-calculus for encoding mobile ambients. Math. Struct. Comput. Sci. 28(2), 202–240 (2018)
Brodo, L., Olarte, C.: Symbolic semantics for multiparty interactions in the link-calculus. In: Steffen, B., Baier, C., van den Brand, M., Eder, J., Hinchey, M., Margaria, T. (eds.) SOFSEM 2017. LNCS, vol. 10139, pp. 62–75. Springer, Cham (2017). https://doi.org/10.1007/978-3-319-51963-0_6
Cardelli, L., Gordon, A.D.: Mobile ambients. Theoret. Comput. Sci. 240(1), 177–213 (2000)
Chiarugi, D., Falaschi, M., Hermith, D., Olarte, C., Torella, L.: Modelling non-markovian dynamics in biochemical reactions. BMC Syst. Biol. 9(S–3), S8 (2015)
Chiarugi, D., Falaschi, M., Olarte, C., Palamidessi, C.: Compositional modelling of signalling pathways in timed concurrent constraint programming. In: Proceedings of ACM BCB 2010, pp. 414–417. ACM, New York (2010)
Corolli, L., Maj, C., Marinia, F., Besozzi, D., Mauri, G.: An excursion in reaction systems: from computer science to biology. Theoret. Comput. Sci. 454, 95–108 (2012)
Falaschi, M., Olarte, C., Palamidessi, C.: Abstract interpretation of temporal concurrent constraint programs. Theory Pract. Logic Program. 15(3), 312–357 (2015)
Mȩski, A., Penczek, W., Rozenberg, G.: Model checking temporal properties of reaction systems. Inf. Sci. 313, 22–42 (2015). https://doi.org/10.1016/j.ins.2015.03.048
Okubo, F., Yokomori, T.: The computational capability of chemical reaction automata. Natural Comput. 15(2), 215–224 (2016). https://doi.org/10.1007/s11047-015-9504-7
Olarte, C.: SiLVer: Symbolic links verifier, December 2018. http://subsell.logic.at/links/links-web/index.html
Olarte, C., Chiarugi, D., Falaschi, M., Hermith, D.: A proof theoretic view of spatial and temporal dependencies in biochemical systems. Theor. Comput. Sci. 641, 25–42 (2016)
Acknowledgments
We thank the anonymous reviewers for their detailed and very useful criticisms and recommendations that helped us to improve our paper.
Author information
Authors and Affiliations
Corresponding author
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2019 Springer Nature Switzerland AG
About this chapter
Cite this chapter
Brodo, L., Bruni, R., Falaschi, M. (2019). Enhancing Reaction Systems: A Process Algebraic Approach. In: Alvim, M., Chatzikokolakis, K., Olarte, C., Valencia, F. (eds) The Art of Modelling Computational Systems: A Journey from Logic and Concurrency to Security and Privacy. Lecture Notes in Computer Science(), vol 11760. Springer, Cham. https://doi.org/10.1007/978-3-030-31175-9_5
Download citation
DOI: https://doi.org/10.1007/978-3-030-31175-9_5
Published:
Publisher Name: Springer, Cham
Print ISBN: 978-3-030-31174-2
Online ISBN: 978-3-030-31175-9
eBook Packages: Computer ScienceComputer Science (R0)