一、树的定义：
1、树：树(Tree)是n(n>=0)个结点的有限集。n=0时称为空树。在任意一颗非空树中：
（1）有且仅有一个特定的称为根(Root)的结点；
（2）当n>1是，其余结点可分为m(m>0)个互不相交的有限集T1,T2,......Tn,其中每一个集合本身又是一棵树，并且称为根的子树 (SubTree).
2、结点的度：结点拥有的子树称为结点的度。度为0的结点称为叶子结点或终端结点，度不为0的结点称为非终端结点或分支结点。除根结点以外，分支结点也称为内部结点。树的度是树内各结点的度的最大值。
3、层次与深度：结点的层次（level）从根考试定义起，根为第一层，根的孩子为第二层。若某结点在第1层，则其子树的根就在第i+1层。其双亲在同一层的结点互为堂兄弟。如下图的D、E、F是堂兄弟，而G、H、I、J也是。是中结点的最大层次称为树的深度（Depth）或高度，当前树的深度为4。
二、二叉树
1、二叉树是一种特殊的树，它的特点是每个结点最多有两个子树（即二叉树的度不能大于2），并且二叉树的子树有左右之分，其次序不能颠倒。
2、一棵深度为k 且有2^k -1 个结点的二叉树称为满二叉树。
3、如果有深度为k 的，有n 个结点的二叉树，如果其每一个结点都与深度为k 的满二叉树中编号从1 至n 的结点一一对应，则称之为完全二叉树。
二叉树性质：
性质1：在二叉树的第i 层上最多有2^(i – 1)个结点（i>=1）。
性质2：深度为k 的二叉树至多有2^i – 1 个结点(k>=1)。
性质3：对如何一颗二叉树T,如果其终端结点数为
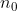
，度为2的结点数为
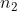
，则
。性质4：具有n个结点的完全二叉树深度为
([x]N表示不大于X的最大整数)。性质5：如果对一颗有n个结点的完全二叉树（其深度为
）的结点按层序编号（从第1层到底
层，每层从左到右），对任意一个结点i（1<=i<=n）有:
1):如果i=1.则结点i是二叉树的根，无双亲；如果i>1，在其双亲是结点[i/2]
2):如果2i>n，则结点i无左孩子（结点i为叶子结点）；否则其左孩子是结点2i
3):如果2i+1>n,则结点i无右孩子；否则其右孩子是结点2i+1
4、二叉树的遍历：(非常重要)
前序遍历:根 左 右;
结果为：A B D G H C E I F
中序遍历:左 根 右;
结果为：G D H B A E I C F
后序遍历:左 右 根;
结果为：G H D B I E F C A
代码如下：
public class BinaryTree {
/**
* BinaryTree 的节点数据结构
*/
private class TreeNode{
private int key = 0;
private String data = null;
private boolean isVisited = false;
private TreeNode leftChild = null;
private TreeNode rightChild = null;
public TreeNode(){}
public TreeNode(int key,String data){
this.key = key;
this.data = data;
this.leftChild = null;
this.rightChild = null;
}
}
//获取根节点
public TreeNode getRoot() {
return root;
}
public void setRoot(TreeNode root) {
this.root = root;
}
//定义根节点
private TreeNode root = null;
public BinaryTree(){
root = new TreeNode(1,"A");
}
/**
* 创建一棵二叉树
*/
public void createBinaryTree(TreeNode root){
TreeNode nodeB = new TreeNode(2,"B");
TreeNode nodeC = new TreeNode(3,"C");
TreeNode nodeD = new TreeNode(4,"D");
TreeNode nodeE = new TreeNode(5,"E");
TreeNode nodeF = new TreeNode(6,"F");
root.leftChild = nodeB;
root.rightChild = nodeC;
nodeB.leftChild = nodeD;
nodeB.rightChild = nodeE;
nodeC.rightChild = nodeF;
}
/**
* 前序遍历
*/
public void preOrder(TreeNode node){
if(node != null){
visited(node);
preOrder(node.leftChild);
preOrder(node.rightChild);
}
}
/**
* 中序遍历
* @param node
*/
public void inOrder(TreeNode node){
if(node != null){
preOrder(node.leftChild);
visited(node);
preOrder(node.rightChild);
}
}
/**
* 后序遍历
* @param node
*/
public void postOrder(TreeNode node){
if(node != null){
preOrder(node.leftChild);
preOrder(node.rightChild);
visited(node);
}
}
/**
* 非递归前序遍历
* @param node
*/
public void nonRecPreOrder(TreeNode node){
Stack<TreeNode> stack = new Stack<>();
TreeNode pNode = node;
while(pNode != null || stack.size()>0){
while(pNode != null){
visited(pNode);
stack.push(pNode);
pNode = pNode.leftChild;
}
if(stack.size()>0){
pNode = stack.pop();
pNode = pNode.rightChild;
}
}
}
/**
* 非递归中序遍历
* @param node
*/
public void nonRecInOrder(TreeNode node){
Stack<TreeNode> stack = new Stack<>();
TreeNode pNode = node;
while(pNode != null || stack.size()>0){
while(pNode != null){
stack.push(pNode);
pNode = pNode.leftChild;
}
if(stack.size()>0){
pNode = stack.pop();
visited(pNode);
pNode = pNode.rightChild;
}
}
}
/**
* 非递归后序遍历
* @param pNode
*/
public void nonRecPostOrder(TreeNode pNode){
Stack<TreeNode> stack = new Stack<>();
TreeNode node = pNode;
while(pNode != null){
//左子树入栈
while(pNode.leftChild != null){
stack.push(pNode);
pNode = pNode.leftChild;
}
//当前节点无右子树或者右子树已输出
while(pNode != null && (pNode.rightChild == null || pNode.rightChild == node)){
visited(pNode);
//记录上一个已输出的节点
node = pNode;
if(!stack.isEmpty()){
pNode = stack.pop();
}else{
return;
}
}
//右子树入栈
stack.push(pNode);
pNode = pNode.rightChild;
}
}
private void visited(TreeNode node) {
node.isVisited = true;
System.out.println(node.data+","+node.key);
}
/**
* 计算树的高度
*/
private int height(TreeNode node){
if(node == null){
return 0;
}else{
int i = height(node.leftChild);
int j = height(node.rightChild);
return (i<j)?j+1:i+1;
}
}
/**
* 计算树的节点数
* @param node
* @return 树的节点数
*/
private int size(TreeNode node){
if(node == null){
return 0;
}else{
return 1+size(node.leftChild)+size(node.rightChild);
}
}
public static void main(String[] args) {
BinaryTree binaryTree = new BinaryTree();
TreeNode root = binaryTree.root;
binaryTree.createBinaryTree(root);
System.out.println(binaryTree.height(root));
System.out.println(binaryTree.size(root));
binaryTree.preOrder(root);
System.out.println("*******");
binaryTree.nonRecPreOrder(root);
System.out.println("*******");
binaryTree.nonRecInOrder(root);
System.out.println("-------------");
binaryTree.nonRecPostOrder(root);
}
}
