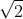Newton迭代法开方（Python）

目录

​​1、概述​​

​​2、Newton迭代法的原理：​​

​​3、案例：用Newton迭代法：​​

​​4、结果​​

---

1、概述

       在对非线性方程根求解时，5次以上的代数方程和超越方程一般没有求根公式，很难或者无法求得其精确解，而在实际应用中要得到满足一定精度的近似解就可以了。

      我们数值解法一般有二分法和Newton迭代法，如图所示。二分法的优点是算法简单，缺点是收敛速度慢，不能求重根。对于本章我们主要讲解Newton迭代法。   

2、Newton迭代法的原理：

3、案例：用Newton迭代法求

：

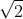
import numpy as np 
def func(x):
    return x**2-2   
def gradfunc(x):
    return 2*x

def computesqrt(f,gradf,x,tol,maxit):
    x0=x
    xk=x0
    k=1
    while (True):
        gf=gradf(x0)
        if gf*gf<10**(-10):
            print("gradient may zeros, please try it again by using another x")
            return -1
        xk=x0-f(x0)/gf
        if (xk-x0)*(xk-x0)<tol*tol:
            print("Tolerance conditon is satisfied: "+str(tol))
            return xk
        if k>maxit:
            print("Max iteration condition is satisfied: "+str(maxit))
            return xk
        print("iter:"+str(k)+","+str(xk))
        x0=xk
        k=k+1


def main():
    x =-0.5
    maxit = 200
    tol = 1.0*10**(-10)
    sqrt2=computesqrt(func,gradfunc,x,tol,maxit)
    print("sqrt2 = "+str(sqrt2))




if __name__ == '__main__':
    main()

4、结果

iter:1,-2.25
iter:2,-1.5694444444444444
iter:3,-1.4218903638151426
iter:4,-1.4142342859400734
iter:5,-1.4142135625249321
iter:6,-1.4142135623730951
Tolerance conditon is satisfied: 1e-10
sqrt2 = -1.414213562373095