hdu5608(积性函数+欧拉函数+杜教筛+莫比乌斯反演)

这个题最显眼的地方是10^9的前缀和和已知因子和的结果了，显然需要杜教筛。。

按照杜教筛的套路，我们设结果为F(n)，那么

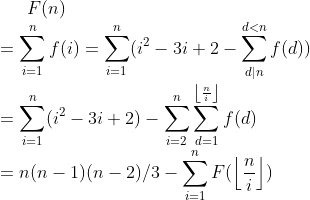
如果直接算空间和时间都承受不住。。需要预处理。。然后又发现一个大麻烦。。

首先可以发现无论是这个函数还是其和函数都不满足积性性质(f(1)!=1)，所以需要转换思路。。。

运用莫比乌斯反演可以得到

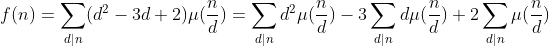
然后发现f(n)就是三个积性函数的和。。而其中第二项其实就是欧拉函数 (推了半天才发现)，第三项其实就是元函数e。。

然后就只剩下第一项了，看来只能用普通积性函数的分析方法分析一波。。令其为g(n)，那么f(n)=g(n)-3φ(n)+2e(n)

而

然后就可以线性筛g(n)了，和φ(n)挺类似的其实。。

然后题目又提示了超过10^6的数据不超过5个，所以干脆直接预处理到10^6，也刚好能形成

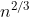
的复杂度。。

预处理完上杜教筛，解决

 

 

 

 

/**
 *　　　　　　  　　┏┓　　 　┏┓
 * 　　　　　  　　┏┛┗━━━━━━━┛┗━━━┓
 * 　　　　　  　　┃　　　　　　　┃ 　
 * 　　　　　  　　┃　　　━　　 　┃
 * 　　　　　  　　┃　＞　　　＜　┃
 * 　　　　　  　　┃　　　　　　　┃
 * 　　　　　  　　┃...　⌒　... 　┃
 * 　　　　　　  　┃              ┃
 * 　　　　　　  　┗━┓          ┏━┛
 * 　　　　　　　　　┃          ┃　Code is far away from bug with the animal protecting　　　　　　　　　　
 * 　　　　　　　　　┃          ┃   神兽保佑,代码无bug
 * 　　　　　　　　　┃          ┃　　　　　　　　　　　
 * 　　　　　　　　　┃          ┃  　　　　　　
 * 　　　　　　　　　┃          ┃
 * 　　　　　　　　　┃          ┃　　　　　　　　　　　
 * 　　　　　　　　　┃          ┗━━━┓
 * 　　　　　　　　　┃              ┣┓
 * 　　　　　　　　　┃              ┏┛
 * 　　　　　　　　　┗┓┓┏━━━━━━━━┳┓┏┛
 * 　　　　　　　　　　┃┫┫       ┃┫┫
 * 　　　　　　　　　　┗┻┛       ┗┻┛
 */
#include<cstdio>
#include<cstring>
#include<algorithm>
#include<iostream>
#include<queue>
#include<cmath>
#include<map>
#include<stack>
#include<set>
#include<bitset>
#define inc(i,l,r) for(int i=l;i<=r;i++)
#define dec(i,l,r) for(int i=l;i>=r;i--)
#define link(x) for(edge *j=h[x];j;j=j->next)
#define mem(a) memset(a,0,sizeof(a))
#define ll long long
#define eps 1e-8
#define succ(x) (1LL<<(x))
#define lowbit(x) (x&(-x))
#define sqr(x) ((x)*(x))
#define mid ((x+y)/2) 
#define NM 1000005
#define nm 10005
#define N 1000005
#define M(x,y) x=max(x,y)
const double pi=acos(-1);
const int inf=1e9+7;
using namespace std;
ll read(){
    ll x=0,f=1;char ch=getchar();
    while(!isdigit(ch)){if(ch=='-')f=-1;ch=getchar();}
    while(isdigit(ch))x=x*10+ch-'0',ch=getchar();
    return f*x;
}
  


int m,prime[NM],tot,phi[NM];
ll _x,f[NM],inv[10],g[NM];

void init(){
    bool v[NM];
    mem(v);
    m=1e6;inv[1]=1;phi[1]=g[1]=1;
    inc(i,2,3)inv[i]=inv[inf%i]*(inf-inf/i)%inf;
    inc(i,2,m){
    if(!v[i])prime[++tot]=i,phi[i]=i-1,g[i]=((ll)i*i-1)%inf;
    inc(j,1,tot){
        if(i*prime[j]>m)break;
        v[i*prime[j]]++;
        if(i%prime[j]==0){
        phi[i*prime[j]]=phi[i]*prime[j];
        g[i*prime[j]]=g[i]*prime[j]%inf*prime[j]%inf;
        break;
        }
        phi[i*prime[j]]=phi[i]*(prime[j]-1);
        g[i*prime[j]]=g[i]*((ll)prime[j]*prime[j]-1)%inf;
    }
    f[i]=g[i]-3*phi[i];f[i]=(f[i-1]+f[i]+inf)%inf;
    }
}

ll v[1005];
ll solve(ll n){
    if(n<=m)return f[n];
    int t=_x/n;
    if(v[t])return v[t];
    v[t]=(ll)n*(n-1)%inf*(n-2)%inf*inv[3]%inf;
    for(ll i=2,j;i<=n;i=j+1){
    j=n/(n/i);
    v[t]=(v[t]-(j-i+1)*solve(n/i)%inf+inf)%inf;
    }
    return v[t];
}


int main(){
    init();
    int _=read();while(_--){
    _x=read();if(_x>m)mem(v);
    printf("%lld\n",solve(_x));
    }
    return 0;
}

 

 

 

function Time Limit: 6000/3000 MS (Java/Others)    Memory Limit: 65536/65536 K (Java/Others) Total Submission(s): 954    Accepted Submission(s): 367   Problem Description There is a function f(x),which is defined on the natural numbers set N,satisfies the following eqaution N2−3N+2=∑d|Nf(d) calulate ∑Ni=1f(i)  mod 109+7.   Input the first line contains a positive integer T,means the number of the test cases. next T lines there is a number N T≤500,N≤109 only 5 test cases has N>106.   Output Tlines,each line contains a number,means the answer to the i-th test case.   Sample Input 1 3   Sample Output 2 $1^2-3*1+2=f(1)=0$ $2^2-3*2+2=f(2)+f(1)=0->f(2)=0$ $3^2-3*3+2=f(3)+f(1)=2->f(3)=2$ $f(1)+f(2)+f(3)=2$   Source ​​BestCoder Round #68 (div.2) ​​   Recommend hujie   |   We have carefully selected several similar problems for you:  ​​6447​​​ ​​6446​​​ ​​6445​​​ ​​6444​​​ ​​6443​​    ​​Statistic​​​ | ​​Submit​​​ | ​​Discuss​​​ | ​​Note​​

​​Home​​​ | ​​Top​​