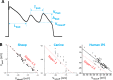
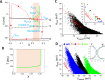
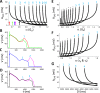
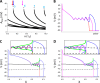
Early afterdepolarizations (EADs) are spontaneous depolarizations during the repolarization phase of an action potential in cardiac myocytes. It is widely known that EADs are promoted by increasing inward currents and/or decreasing outward currents, a condition called reduced repolarization reserve. Recent studies based on bifurcation theories show that EADs are caused by a dual Hopf-homoclinic bifurcation, bringing in further mechanistic insights into the genesis and dynamics of EADs. In this study, we investigated the EAD properties, such as the EAD amplitude, the inter-EAD interval, and the latency of the first EAD, and their major determinants. We first made predictions based on the bifurcation theory and then validated them in physiologically more detailed action potential models. These properties were investigated by varying one parameter at a time or using parameter sets randomly drawn from assigned intervals. The theoretical and simulation results were compared with experimental data from the literature. Our major findings are that the EAD amplitude and takeoff potential exhibit a negative linear correlation; the inter-EAD interval is insensitive to the maximum ionic current conductance but mainly determined by the kinetics of ICa,L and the dual Hopf-homoclinic bifurcation; and both inter-EAD interval and latency vary largely from model to model. Most of the model results generally agree with experimental observations in isolated ventricular myocytes. However, a major discrepancy between modeling results and experimental observations is that the inter-EAD intervals observed in experiments are mainly between 200 and 500 ms, irrespective of species, while those of the mathematical models exhibit a much wider range with some models exhibiting inter-EAD intervals less than 100 ms. Our simulations show that the cause of this discrepancy is likely due to the difference in ICa,L recovery properties in different mathematical models, which needs to be addressed in future action potential model development.