Homeostatic role of heterosynaptic plasticity: models and experiments
- PMID: 26217218
- PMCID: PMC4500102
- DOI: 10.3389/fncom.2015.00089
Homeostatic role of heterosynaptic plasticity: models and experiments
Abstract
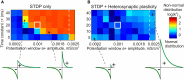
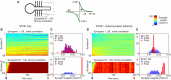
Homosynaptic Hebbian-type plasticity provides a cellular mechanism of learning and refinement of connectivity during development in a variety of biological systems. In this review we argue that a complimentary form of plasticity-heterosynaptic plasticity-represents a necessary cellular component for homeostatic regulation of synaptic weights and neuronal activity. The required properties of a homeostatic mechanism which acutely constrains the runaway dynamics imposed by Hebbian associative plasticity have been well-articulated by theoretical and modeling studies. Such mechanism(s) should robustly support the stability of operation of neuronal networks and synaptic competition, include changes at non-active synapses, and operate on a similar time scale to Hebbian-type plasticity. The experimentally observed properties of heterosynaptic plasticity have introduced it as a strong candidate to fulfill this homeostatic role. Subsequent modeling studies which incorporate heterosynaptic plasticity into model neurons with Hebbian synapses (utilizing an STDP learning rule) have confirmed its ability to robustly provide stability and competition. In contrast, properties of homeostatic synaptic scaling, which is triggered by extreme and long lasting (hours and days) changes of neuronal activity, do not fit two crucial requirements for a hypothetical homeostatic mechanism needed to provide stability of operation in the face of on-going synaptic changes driven by Hebbian-type learning rules. Both the trigger and the time scale of homeostatic synaptic scaling are fundamentally different from those of the Hebbian-type plasticity. We conclude that heterosynaptic plasticity, which is triggered by the same episodes of strong postsynaptic activity and operates on the same time scale as Hebbian-type associative plasticity, is ideally suited to serve a homeostatic role during on-going synaptic plasticity.
Keywords: Hebbian plasticity; STDP; heterosynaptic plasticity; homeostasis; homosynaptic plasticity; runaway dynamics; synaptic plasticity.
Figures

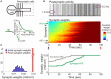

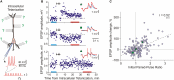
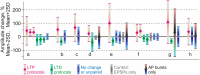
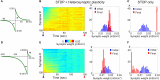
Similar articles
-
Partial Breakdown of Input Specificity of STDP at Individual Synapses Promotes New Learning.J Neurosci. 2016 Aug 24;36(34):8842-55. doi: 10.1523/JNEUROSCI.0552-16.2016. J Neurosci. 2016. PMID: 27559167 Free PMC article.
-
Heterosynaptic plasticity: multiple mechanisms and multiple roles.Neuroscientist. 2014 Oct;20(5):483-98. doi: 10.1177/1073858414529829. Epub 2014 Apr 11. Neuroscientist. 2014. PMID: 24727248 Free PMC article. Review.
-
Adenosine Shifts Plasticity Regimes between Associative and Homeostatic by Modulating Heterosynaptic Changes.J Neurosci. 2017 Feb 8;37(6):1439-1452. doi: 10.1523/JNEUROSCI.2984-16.2016. Epub 2016 Dec 27. J Neurosci. 2017. PMID: 28028196 Free PMC article.
-
Heterosynaptic plasticity-induced modulation of synapses.J Physiol Sci. 2023 Dec 6;73(1):33. doi: 10.1186/s12576-023-00893-1. J Physiol Sci. 2023. PMID: 38057729 Free PMC article. Review.
-
Heterosynaptic plasticity prevents runaway synaptic dynamics.J Neurosci. 2013 Oct 2;33(40):15915-29. doi: 10.1523/JNEUROSCI.5088-12.2013. J Neurosci. 2013. PMID: 24089497 Free PMC article.
Cited by
-
Enhanced Non-Associative Long-Term Potentiation in Immature Granule Cells in the Dentate Gyrus of Adult Rats.Front Synaptic Neurosci. 2022 May 30;14:889947. doi: 10.3389/fnsyn.2022.889947. eCollection 2022. Front Synaptic Neurosci. 2022. PMID: 35711669 Free PMC article.
-
Memory destabilization during reconsolidation: a consequence of homeostatic plasticity?Learn Mem. 2021 Sep 15;28(10):371-389. doi: 10.1101/lm.053418.121. Print 2021 Oct. Learn Mem. 2021. PMID: 34526382 Free PMC article.
-
Long-Term Homeostatic Properties Complementary to Hebbian Rules in CuPc-Based Multifunctional Memristor.Sci Rep. 2016 Oct 20;6:35273. doi: 10.1038/srep35273. Sci Rep. 2016. PMID: 27762316 Free PMC article.
-
Stability and learning in excitatory synapses by nonlinear inhibitory plasticity.PLoS Comput Biol. 2022 Dec 2;18(12):e1010682. doi: 10.1371/journal.pcbi.1010682. eCollection 2022 Dec. PLoS Comput Biol. 2022. PMID: 36459503 Free PMC article.
-
Synaptic Plasticity in Cortical Inhibitory Neurons: What Mechanisms May Help to Balance Synaptic Weight Changes?Front Cell Neurosci. 2020 Sep 4;14:204. doi: 10.3389/fncel.2020.00204. eCollection 2020. Front Cell Neurosci. 2020. PMID: 33100968 Free PMC article. Review.
References
-
- Aitkin L. M., Anderson D. J., Brugge J. F. (1970). Tonotopic organization and discharge characteristics of single neurons in nuclei of the lateral lemniscus of the cat. J. Neurophysiol. 33, 421–440. - PubMed
Publication types
Grants and funding
LinkOut - more resources
Full Text Sources
Other Literature Sources

